Elongated pentagonal orthobicupola

Last updated
Elongated pentagonal orthobicupola
Elongated pentagonal orthobicupola.png
Type Johnson
J37 – J38 – J39
Faces 10 triangles
2x5+10 squares
2 pentagons
Edges 60
Vertices 30
Vertex configuration 20(3.43)
10(3.4.5.4)
Symmetry group D5h
Dual polyhedron -
Properties convex
Net
Johnson solid 38 net.png

In geometry, the elongated pentagonal orthobicupola or cantellated pentagonal prism is one of the Johnson solids (J38). [1] As the name suggests, it can be constructed by elongating a pentagonal orthobicupola (J30) by inserting a decagonal prism between its two congruent halves. Rotating one of the cupolae through 36 degrees before inserting the prism yields an elongated pentagonal gyrobicupola (J39).

Contents

A Johnson solid is one of 92 strictly convex polyhedra that is composed of regular polygon faces but are not uniform polyhedra (that is, they are not Platonic solids , Archimedean solids , prisms , or antiprisms ). They were named by Norman Johnson , who first listed these polyhedra in 1966. [2]

Formulae

The following formulae for volume and surface area can be used if all faces are regular, with edge length a: [3]

Related Research Articles

<span class="mw-page-title-main">Elongated pentagonal pyramid</span> 9th Johnson solid (11 faces)

In geometry, the elongated pentagonal pyramid is one of the Johnson solids. As the name suggests, it can be constructed by elongating a pentagonal pyramid by attaching a pentagonal prism to its base.

<span class="mw-page-title-main">Elongated square cupola</span> 19th Johnson solid

In geometry, the elongated square cupola is one of the Johnson solids. As the name suggests, it can be constructed by elongating a square cupola by attaching an octagonal prism to its base. The solid can be seen as a rhombicuboctahedron with its "lid" removed.

<span class="mw-page-title-main">Elongated pentagonal rotunda</span>

In geometry, the elongated pentagonal rotunda is one of the Johnson solids (J21). As the name suggests, it can be constructed by elongating a pentagonal rotunda (J6) by attaching a decagonal prism to its base. It can also be seen as an elongated pentagonal orthobirotunda (J42) with one pentagonal rotunda removed.

<span class="mw-page-title-main">Square gyrobicupola</span> 29th Johnson solid; 2 square cupolae joined base-to-base

In geometry, the square gyrobicupola is one of the Johnson solids. Like the square orthobicupola, it can be obtained by joining two square cupolae along their bases. The difference is that in this solid, the two halves are rotated 45 degrees with respect to one another.

<span class="mw-page-title-main">Elongated pentagonal gyrobirotunda</span> 43rd Johnson solid

In geometry, the elongated pentagonal gyrobirotunda is one of the Johnson solids. As the name suggests, it can be constructed by elongating a "pentagonal gyrobirotunda," or icosidodecahedron, by inserting a decagonal prism between its congruent halves. Rotating one of the pentagonal rotundae through 36 degrees before inserting the prism yields an elongated pentagonal orthobirotunda.

<span class="mw-page-title-main">Elongated pentagonal orthobirotunda</span> 42nd Johnson solid

In geometry, the elongated pentagonal orthobirotunda is one of the Johnson solids. Its Conway polyhedron notation is at5jP5. As the name suggests, it can be constructed by elongating a pentagonal orthobirotunda by inserting a decagonal prism between its congruent halves. Rotating one of the pentagonal rotundae through 36 degrees before inserting the prism yields the elongated pentagonal gyrobirotunda.

<span class="mw-page-title-main">Gyroelongated pentagonal birotunda</span> 48th Johnson solid

In geometry, the gyroelongated pentagonal birotunda is one of the Johnson solids. As the name suggests, it can be constructed by gyroelongating a pentagonal birotunda by inserting a decagonal antiprism between its two halves.

<span class="mw-page-title-main">Elongated triangular bipyramid</span> 14th Johnson solid; triangular prism capped with tetrahedra

In geometry, the elongated triangular bipyramid or triakis triangular prism is one of the Johnson solids, convex polyhedra whose faces are regular polygons. As the name suggests, it can be constructed by elongating a triangular bipyramid by inserting a triangular prism between its congruent halves.

<span class="mw-page-title-main">Elongated pentagonal cupola</span> 20th Johnson solid

In geometry, the elongated pentagonal cupola is one of the Johnson solids. As the name suggests, it can be constructed by elongating a pentagonal cupola by attaching a decagonal prism to its base. The solid can also be seen as an elongated pentagonal orthobicupola with its "lid" removed.

<span class="mw-page-title-main">Pentagonal orthobicupola</span> 30th Johnson solid; 2 pentagonal cupolae joined base-to-base

In geometry, the pentagonal orthobicupola is one of the Johnson solids. As the name suggests, it can be constructed by joining two pentagonal cupolae along their decagonal bases, matching like faces. A 36-degree rotation of one cupola before the joining yields a pentagonal gyrobicupola.

<span class="mw-page-title-main">Pentagonal gyrobicupola</span> 31st Johnson solid; 2 pentagonal cupolae joined base-to-base

In geometry, the pentagonal gyrobicupola is one of the Johnson solids. Like the pentagonal orthobicupola, it can be obtained by joining two pentagonal cupolae along their bases. The difference is that in this solid, the two halves are rotated 36 degrees with respect to one another.

<span class="mw-page-title-main">Elongated pentagonal gyrobicupola</span> 39th Johnson solid

In geometry, the elongated pentagonal gyrobicupola is one of the Johnson solids. As the name suggests, it can be constructed by elongating a pentagonal gyrobicupola by inserting a decagonal prism between its congruent halves. Rotating one of the pentagonal cupolae through 36 degrees before inserting the prism yields an elongated pentagonal orthobicupola.

<span class="mw-page-title-main">Elongated triangular cupola</span> 18th Johnson solid

In geometry, the elongated triangular cupola is one of the Johnson solids. As the name suggests, it can be constructed by elongating a triangular cupola by attaching a hexagonal prism to its base.

<span class="mw-page-title-main">Triangular orthobicupola</span> 27th Johnson solid; 2 triangular cupolae joined base-to-base

In geometry, the triangular orthobicupola is one of the Johnson solids. As the name suggests, it can be constructed by attaching two triangular cupolas along their bases. It has an equal number of squares and triangles at each vertex; however, it is not vertex-transitive. It is also called an anticuboctahedron, twisted cuboctahedron or disheptahedron. It is also a canonical polyhedron.

<span class="mw-page-title-main">Pentagonal orthocupolarotunda</span> 32nd Johnson solid; pentagonal cupola and rotunda joined base-to-base

In geometry, the pentagonal orthocupolarotunda is one of the Johnson solids. As the name suggests, it can be constructed by joining a pentagonal cupola and a pentagonal rotunda along their decagonal bases, matching the pentagonal faces. A 36-degree rotation of one of the halves before the joining yields a pentagonal gyrocupolarotunda.

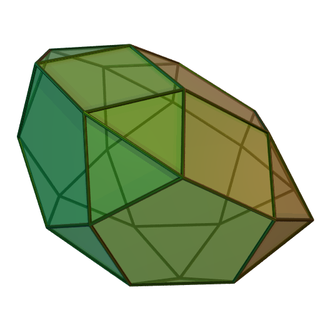
<span class="mw-page-title-main">Pentagonal gyrocupolarotunda</span> 33rd Johnson solid; pentagonal cupola and rotunda joined base-to-base

In geometry, the pentagonal gyrocupolarotunda is one of the Johnson solids. Like the pentagonal orthocupolarotunda, it can be constructed by joining a pentagonal cupola and a pentagonal rotunda along their decagonal bases. The difference is that in this solid, the two halves are rotated 36 degrees with respect to one another.

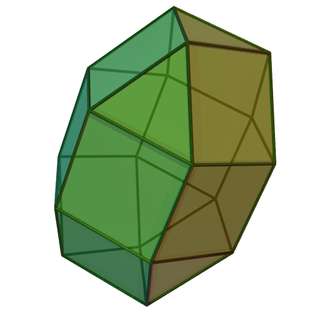
<span class="mw-page-title-main">Elongated triangular gyrobicupola</span> 36th Johnson solid

In geometry, the elongated triangular gyrobicupola is one of the Johnson solids. As the name suggests, it can be constructed by elongating a "triangular gyrobicupola," or cuboctahedron, by inserting a hexagonal prism between its two halves, which are congruent triangular cupolae. Rotating one of the cupolae through 60 degrees before the elongation yields the triangular orthobicupola.

<span class="mw-page-title-main">Gyroelongated triangular bicupola</span> 44th Johnson solid

In geometry, the gyroelongated triangular bicupola is one of the Johnson solids. As the name suggests, it can be constructed by gyroelongating a triangular bicupola by inserting a hexagonal antiprism between its congruent halves.

<span class="mw-page-title-main">Elongated pentagonal gyrocupolarotunda</span> 41st Johnson solid

In geometry, the elongated pentagonal gyrocupolarotunda is one of the Johnson solids. As the name suggests, it can be constructed by elongating a pentagonal gyrocupolarotunda by inserting a decagonal prism between its halves. Rotating either the pentagonal cupola or the pentagonal rotunda through 36 degrees before inserting the prism yields an elongated pentagonal orthocupolarotunda.

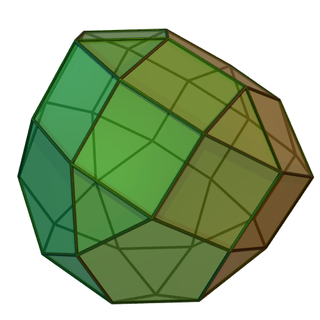
<span class="mw-page-title-main">Elongated pentagonal orthocupolarotunda</span> 40th Johnson solid

In geometry, the elongated pentagonal orthocupolarotunda is one of the Johnson solids. As the name suggests, it can be constructed by elongating a pentagonal orthocupolarotunda by inserting a decagonal prism between its halves. Rotating either the cupola or the rotunda through 36 degrees before inserting the prism yields an elongated pentagonal gyrocupolarotunda.

References

  1. ↑ Weisstein, Eric W. "Elongated Pentagonal Orthobicupola". mathworld.wolfram.com. Retrieved 2023-10-09.
  2. ↑ Johnson, Norman W. (1966), "Convex polyhedra with regular faces", Canadian Journal of Mathematics , 18: 169–200, doi:10.4153/cjm-1966-021-8, MR   0185507, Zbl   0132.14603 .
  3. ↑ Stephen Wolfram, "Elongated pentagonal orthobicupola" from Wolfram Alpha. Retrieved July 25, 2010.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.