Triangular hebesphenorotunda

Triangular hebesphenorotunda
Triangular hebesphenorotunda
Type	Johnson ; J91 – J92 – J1
Faces	13 triangles ; 3 squares ; 3 pentagons ; 1 hexagon
Edges	36
Vertices	18
Vertex configuration	3(33.5) ; 6(3.4.3.5) ; 3(3.5.3.5) ; 2.3(32.4.6)
Symmetry group	C3v
Dual polyhedron	-
Properties	convex, elementary
Net

Last updated September 20, 2024

In geometry, the triangular hebesphenorotunda is a Johnson solid with 13 equilateral triangles, 3 squares, 3 regular pentagons, and 1 regular hexagon, making the total of its faces is 20.

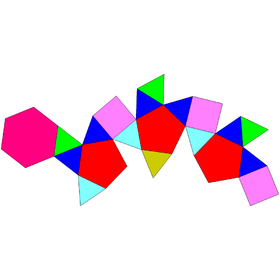
Properties

The triangular hebesphenorotunda is named by Johnson (1966), with the prefix hebespheno- referring to a blunt wedge-like complex formed by three adjacent lunes—a figure where two equilateral triangles are attached at the opposite sides of a square. The suffix (triangular) -rotunda refers to the complex of three equilateral triangles and three regular pentagons surrounding another equilateral triangle, which bears a structural resemblance to the pentagonal rotunda.^[1] Therefore, the triangular hebesphenorotunda has 20 faces: 13 equilateral triangles, 3 squares, 3 regular pentagons, and 1 regular hexagon.^[2] The faces are all regular polygons, categorizing the triangular hebesphenorotunda as the Johnson solid, enumerated the last one $J_{92}$ .^[3] It is elementary polyhedra, meaning that it cannot be separated by a plane into two small regular-faced polyhedra.^[4]

The surface area of a triangular hebesphenorotunda of edge length $a$ as:^[2] $A=\left(3+{\frac {1}{4}}{\sqrt {1308+90{\sqrt {5}}+114{\sqrt {75+30{\sqrt {5}}}}}}\right)a^{2}\approx 16.389a^{2},$ and its volume as:^[2] $V={\frac {1}{6}}\left(15+7{\sqrt {5}}\right)a^{3}\approx 5.10875a^{3}.$

Cartesian coordinates

The triangular hebesphenorotunda with edge length ${\sqrt {5}}-1$ can be constructed by the union of the orbits of the Cartesian coordinates: ${\begin{aligned}\left(0,-{\frac {2}{\tau {\sqrt {3}}}},{\frac {2\tau }{\sqrt {3}}}\right),\qquad &\left(\tau ,{\frac {1}{{\sqrt {3}}\tau ^{2}}},{\frac {2}{\sqrt {3}}}\right)\\\left(\tau ,-{\frac {\tau }{\sqrt {3}}},{\frac {2}{{\sqrt {3}}\tau }}\right),\qquad &\left({\frac {2}{\tau }},0,0\right),\end{aligned}}$ under the action of the group generated by rotation by 120° around the z-axis and the reflection about the yz-plane. Here, $\tau$ denotes the golden ratio.^[5]

Related Research Articles

<span class="mw-page-title-main">Regular icosahedron</span> Convex polyhedron with 20 triangular faces

In geometry, the regular icosahedron is a convex polyhedron that can be constructed from pentagonal antiprism by attaching two pentagonal pyramids with regular faces to each of its pentagonal faces, or by putting points onto the cube. The resulting polyhedron has 20 equilateral triangles as its faces, 30 edges, and 12 vertices. It is an example of a Platonic solid and of a deltahedron. The icosahedral graph represents the skeleton of a regular icosahedron.

<span class="mw-page-title-main">Icosidodecahedron</span> Archimedean solid with 32 faces

In geometry, an icosidodecahedron or pentagonal gyrobirotunda is a polyhedron with twenty (icosi) triangular faces and twelve (dodeca) pentagonal faces. An icosidodecahedron has 30 identical vertices, with two triangles and two pentagons meeting at each, and 60 identical edges, each separating a triangle from a pentagon. As such, it is one of the Archimedean solids and more particularly, a quasiregular polyhedron.

In geometry, an octahedron is a polyhedron with eight faces. One special case is the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex. Regular octahedra occur in nature as crystal structures. Many types of irregular octahedra also exist, including both convex and non-convex shapes.

In geometry, the truncated dodecahedron is an Archimedean solid. It has 12 regular decagonal faces, 20 regular triangular faces, 60 vertices and 90 edges.

In geometry, the gyroelongated square bipyramid is a polyhedron with 16 triangular faces. it can be constructed from a square antiprism by attaching two equilateral square pyramids to each of its square faces. The same shape is also called hexakaidecadeltahedron, heccaidecadeltahedron, or tetrakis square antiprism; these last names mean a polyhedron with 16 triangular faces. It is an example of deltahedron, and of a Johnson solid.

The triaugmented triangular prism, in geometry, is a convex polyhedron with 14 equilateral triangles as its faces. It can be constructed from a triangular prism by attaching equilateral square pyramids to each of its three square faces. The same shape is also called the tetrakis triangular prism, tricapped trigonal prism, tetracaidecadeltahedron, or tetrakaidecadeltahedron; these last names mean a polyhedron with 14 triangular faces. It is an example of a deltahedron, composite polyhedron, and Johnson solid.

In geometry, a square pyramid is a pyramid with a square base, having a total of five faces. If the apex of the pyramid is directly above the center of the square, it is a right square pyramid with four isosceles triangles; otherwise, it is an oblique square pyramid. When all of the pyramid's edges are equal in length, its triangles are all equilateral. It is called an equilateral square pyramid, an example of a Johnson solid.

In geometry, the triangular cupola is the cupola with hexagon as its base and triangle as its top. If the edges are equal in length, the triangular cupola is the Johnson solid. It can be seen as half a cuboctahedron. The triangular cupola can be applied to construct many polyhedrons.

<span class="mw-page-title-main">Bilunabirotunda</span> 91st Johnson solid (14 faces)

In geometry, the bilunabirotunda is a Johnson solid with faces of 8 equilateral triangles, 2 squares, and 4 regular pentagons.

In geometry, the elongated triangular pyramid is one of the Johnson solids. As the name suggests, it can be constructed by elongating a tetrahedron by attaching a triangular prism to its base. Like any elongated pyramid, the resulting solid is topologically self-dual.

In geometry, the elongated triangular bipyramid or triakis triangular prism a polyhedron constructed from a triangular prism by attaching two tetrahedrons to its bases. It is an example of Johnson solid.

In geometry, the elongated pentagonal bipyramid is a polyhedron constructed by attaching two pentagonal pyramids onto the base of a pentagonal prism. It is an example of Johnson solid.

In geometry, the augmented triangular prism is a polyhedron constructed by attaching an equilateral square pyramid onto the square face of a triangular prism. As a result, it is an example of Johnson solid. It can be visualized as the chemical compound, known as capped trigonal prismatic molecular geometry.

In geometry, the biaugmented triangular prism is a polyhedron constructed from a triangular prism by attaching two equilateral square pyramids onto two of its square faces. It is an example of Johnson solid. It can be found in stereochemistry in bicapped trigonal prismatic molecular geometry.

In geometry, the augmented pentagonal prism is a polyhedron that can be constructed by attaching an equilateral square pyramid onto the square face of pentagonal prism. It is an example of Johnson solid.

In geometry, the biaugmented pentagonal prism is a polyhedron constructed from a pentagonal prism by attaching two equilateral square pyramids onto each of its square faces. It is an example of Johnson solid.

In geometry, the augmented hexagonal prism is one of the Johnson solids. As the name suggests, it can be constructed by augmenting a hexagonal prism by attaching a square pyramid to one of its equatorial faces. When two or three such pyramids are attached, the result may be a parabiaugmented hexagonal prism, a metabiaugmented hexagonal prism, or a triaugmented hexagonal prism.

In geometry, the gyroelongated triangular cupola is one of the Johnson solids (J₂₂). It can be constructed by attaching a hexagonal antiprism to the base of a triangular cupola (J₃). This is called "gyroelongation", which means that an antiprism is joined to the base of a solid, or between the bases of more than one solid.

In geometry, the elongated triangular orthobicupola is a polyhedron constructed by attaching two regular triangular cupola into the base of a regular hexagonal prism. It is an example of Johnson solid.

In geometry, the elongated triangular gyrobicupola is a polyhedron constructed by attaching two regular triangular cupolas to the base of a regular hexagonal prism, with one of them rotated in $. It is an example of Johnson solid.$

References

↑ Johnson, N. W. (1966), "Convex polyhedra with regular faces", Canadian Journal of Mathematics , 18: 169–200, doi: 10.4153/cjm-1966-021-8 , MR 0185507, S2CID 122006114, Zbl 0132.14603 .
1 2 3 Berman, M. (1971), "Regular-faced convex polyhedra", Journal of the Franklin Institute, 291 (5): 329–352, doi:10.1016/0016-0032(71)90071-8, MR 0290245 .
↑ Francis, D. (August 2013), "Johnson solids & their acronyms", Word Ways, 46 (3): 177.
↑ Cromwell, P. R. (1997), Polyhedra, Cambridge University Press, p. 86–87, 89, ISBN 978-0-521-66405-9 .
↑ Timofeenko, A. V. (2009), "The non-Platonic and non-Archimedean noncomposite polyhedra", Journal of Mathematical Science, 162 (5): 717, doi:10.1007/s10958-009-9655-0, S2CID 120114341 .

External links

Weisstein, Eric W., "Triangular hebesphenorotunda" ("Johnson solid") at MathWorld .

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[johnson-1] Johnson, N. W. (1966), "Convex polyhedra with regular faces", Canadian Journal of Mathematics , 18: 169–200, doi: 10.4153/cjm-1966-021-8 , MR 0185507, S2CID 122006114, Zbl 0132.14603 .

[berman-2] 1 2 3 Berman, M. (1971), "Regular-faced convex polyhedra", Journal of the Franklin Institute, 291 (5): 329–352, doi:10.1016/0016-0032(71)90071-8, MR 0290245 .

[francis-3] Francis, D. (August 2013), "Johnson solids & their acronyms", Word Ways, 46 (3): 177.

[cromwell-4] Cromwell, P. R. (1997), Polyhedra, Cambridge University Press, p. 86–87, 89, ISBN 978-0-521-66405-9 .

[timofeenko-5] Timofeenko, A. V. (2009), "The non-Platonic and non-Archimedean noncomposite polyhedra", Journal of Mathematical Science, 162 (5): 717, doi:10.1007/s10958-009-9655-0, S2CID 120114341 .

[1]

[2]

[3]

[4]

[5]

v t e Johnson solids
Pyramids, cupolae and rotundae	square pyramid pentagonal pyramid triangular cupola square cupola pentagonal cupola pentagonal rotunda
Modified pyramids	elongated triangular pyramid elongated square pyramid elongated pentagonal pyramid gyroelongated square pyramid gyroelongated pentagonal pyramid triangular bipyramid pentagonal bipyramid elongated triangular bipyramid elongated square bipyramid elongated pentagonal bipyramid gyroelongated square bipyramid
Modified cupolae and rotundae	elongated triangular cupola elongated square cupola elongated pentagonal cupola elongated pentagonal rotunda gyroelongated triangular cupola gyroelongated square cupola gyroelongated pentagonal cupola gyroelongated pentagonal rotunda gyrobifastigium triangular orthobicupola square orthobicupola square gyrobicupola pentagonal orthobicupola pentagonal gyrobicupola pentagonal orthocupolarotunda pentagonal gyrocupolarotunda pentagonal orthobirotunda elongated triangular orthobicupola elongated triangular gyrobicupola elongated square gyrobicupola elongated pentagonal orthobicupola elongated pentagonal gyrobicupola elongated pentagonal orthocupolarotunda elongated pentagonal gyrocupolarotunda elongated pentagonal orthobirotunda elongated pentagonal gyrobirotunda gyroelongated triangular bicupola gyroelongated square bicupola gyroelongated pentagonal bicupola gyroelongated pentagonal cupolarotunda gyroelongated pentagonal birotunda
Augmented prisms	augmented triangular prism biaugmented triangular prism triaugmented triangular prism augmented pentagonal prism biaugmented pentagonal prism augmented hexagonal prism parabiaugmented hexagonal prism metabiaugmented hexagonal prism triaugmented hexagonal prism
Modified Platonic solids	augmented dodecahedron parabiaugmented dodecahedron metabiaugmented dodecahedron triaugmented dodecahedron metabidiminished icosahedron tridiminished icosahedron augmented tridiminished icosahedron
Modified Archimedean solids	augmented truncated tetrahedron augmented truncated cube biaugmented truncated cube augmented truncated dodecahedron parabiaugmented truncated dodecahedron metabiaugmented truncated dodecahedron triaugmented truncated dodecahedron gyrate rhombicosidodecahedron parabigyrate rhombicosidodecahedron metabigyrate rhombicosidodecahedron trigyrate rhombicosidodecahedron diminished rhombicosidodecahedron paragyrate diminished rhombicosidodecahedron metagyrate diminished rhombicosidodecahedron bigyrate diminished rhombicosidodecahedron parabidiminished rhombicosidodecahedron metabidiminished rhombicosidodecahedron gyrate bidiminished rhombicosidodecahedron tridiminished rhombicosidodecahedron
Other elementary solids	snub disphenoid snub square antiprism sphenocorona augmented sphenocorona sphenomegacorona hebesphenomegacorona disphenocingulum bilunabirotunda triangular hebesphenorotunda
(See also List of Johnson solids, a sortable table)

Triangular hebesphenorotunda

Type	Johnson $J 91 - J 92 - J 1$
Faces	13 triangles 3 squares 3 pentagons 1 hexagon
Edges	36
Vertices	18
Vertex configuration	$3(3 3 .5) 6(3.4.3.5) 3(3.5.3.5) 2.3(3 2 .4.6)$
Symmetry group	$C 3v$
Dual polyhedron	-
Properties	convex, elementary
Net