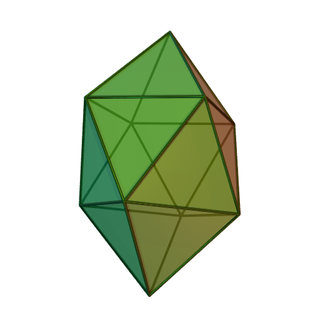
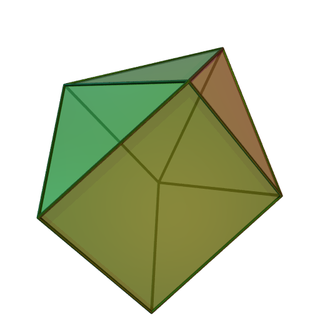
In geometry, the gyroelongated square bipyramid, heccaidecadeltahedron, or tetrakis square antiprism is one of the Johnson solids. As the name suggests, it can be constructed by gyroelongating an octahedron by inserting a square antiprism between its congruent halves. It is one of the eight strictly-convex deltahedra.

In geometry, the augmented tridiminished icosahedron is one of the Johnson solids. It can be obtained by joining a tetrahedron to another Johnson solid, the tridiminished icosahedron.
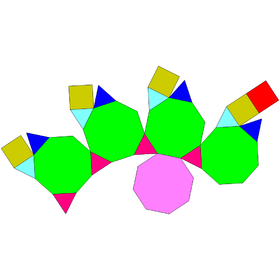
In geometry, the bilunabirotunda is one of the Johnson solids. A Johnson solid is one of 92 strictly convex polyhedra that is composed of regular polygon faces but are not uniform polyhedra. They were named by Norman Johnson, who first listed these polyhedra in 1966.
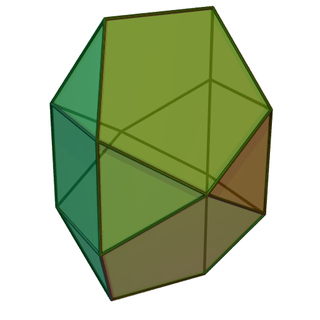
In geometry, the augmented triangular prism is one of the Johnson solids. As the name suggests, it can be constructed by augmenting a triangular prism by attaching a square pyramid to one of its equatorial faces. The resulting solid bears a superficial resemblance to the gyrobifastigium, the difference being that the latter is constructed by attaching a second triangular prism, rather than a square pyramid.

In geometry, the biaugmented triangular prism is one of the Johnson solids. As the name suggests, it can be constructed by augmenting a triangular prism by attaching square pyramids to two of its equatorial faces.

In geometry, the augmented pentagonal prism is one of the Johnson solids. As the name suggests, it can be constructed by augmenting a pentagonal prism by attaching a square pyramid to one of its equatorial faces.

In geometry, the biaugmented pentagonal prism is one of the Johnson solids. As the name suggests, it can be constructed by doubly augmenting a pentagonal prism by attaching square pyramids to two of its nonadjacent equatorial faces.

In geometry, the augmented hexagonal prism is one of the Johnson solids. As the name suggests, it can be constructed by augmenting a hexagonal prism by attaching a square pyramid to one of its equatorial faces. When two or three such pyramids are attached, the result may be a parabiaugmented hexagonal prism, a metabiaugmented hexagonal prism, or a triaugmented hexagonal prism.

In geometry, the parabiaugmented hexagonal prism is one of the Johnson solids. As the name suggests, it can be constructed by doubly augmenting a hexagonal prism by attaching square pyramids to two of its nonadjacent, parallel (opposite) equatorial faces. Attaching the pyramids to nonadjacent, nonparallel equatorial faces yields a metabiaugmented hexagonal prism.

In geometry, the metabiaugmented hexagonal prism is one of the Johnson solids. As the name suggests, it can be constructed by doubly augmenting a hexagonal prism by attaching square pyramids to two of its nonadjacent, nonparallel equatorial faces. Attaching the pyramids to opposite equatorial faces yields a parabiaugmented hexagonal prism.

In geometry, the triaugmented hexagonal prism is one of the Johnson solids. As the name suggests, it can be constructed by triply augmenting a hexagonal prism by attaching square pyramids to three of its nonadjacent equatorial faces.

In geometry, the augmented dodecahedron is one of the Johnson solids, consisting of a dodecahedron with a pentagonal pyramid attached to one of the faces. When two or three such pyramids are attached, the result may be a parabiaugmented dodecahedron, a metabiaugmented dodecahedron, or a triaugmented dodecahedron.

In geometry, the parabiaugmented dodecahedron is one of the Johnson solids. It can be seen as a dodecahedron with two pentagonal pyramids attached to opposite faces. When pyramids are attached to a dodecahedron in other ways, they may result in an augmented dodecahedron, a metabiaugmented dodecahedron, a triaugmented dodecahedron, or even a pentakis dodecahedron if the faces are made to be irregular.

In geometry, the triaugmented dodecahedron is one of the Johnson solids. It can be seen as a dodecahedron with three pentagonal pyramids attached to nonadjacent faces. When pyramids are attached to a dodecahedron in other ways, they may result in an augmented dodecahedron, a parabiaugmented dodecahedron, a metabiaugmented dodecahedron, or even a pentakis dodecahedron if the faces are made to be irregular.

In geometry, the augmented truncated tetrahedron is one of the Johnson solids. It is created by attaching a triangular cupola to one hexagonal face of a truncated tetrahedron.

In geometry, the biaugmented truncated cube is one of the Johnson solids. As its name suggests, it is created by attaching two square cupolas onto two parallel octagonal faces of a truncated cube.
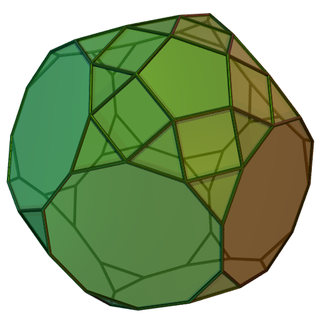
In geometry, the augmented truncated dodecahedron is one of the Johnson solids. As its name suggests, it is created by attaching a pentagonal cupola onto one decagonal face of a truncated dodecahedron.

In geometry, the parabiaugmented truncated dodecahedron is one of the Johnson solids. As its name suggests, it is created by attaching two pentagonal cupolas onto two parallel decagonal faces of a truncated dodecahedron.
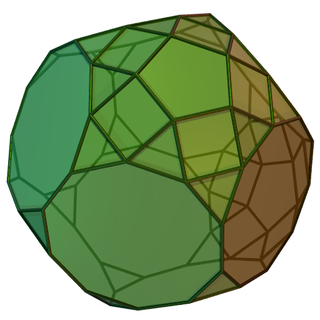
In geometry, the metabiaugmented truncated dodecahedron is one of the Johnson solids. As its name suggests, it is created by attaching two pentagonal cupolas onto two nonadjacent, nonparallel decagonal faces of a truncated dodecahedron.

In geometry, the triaugmented truncated dodecahedron is one of the Johnson solids ; of them, it has the greatest volume in proportion to the cube of the side length. As its name suggests, it is created by attaching three pentagonal cupolas onto three nonadjacent decagonal faces of a truncated dodecahedron.