In geometry, a Johnson solid is a strictly convex polyhedron each face of which is a regular polygon. There is no requirement that each face must be the same polygon, or that the same polygons join around each vertex. An example of a Johnson solid is the square-based pyramid with equilateral sides ; it has 1 square face and 4 triangular faces. Some authors require that the solid not be uniform before they refer to it as a "Johnson solid".

In geometry, an octahedron is a polyhedron with eight faces. The term is most commonly used to refer to the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex.
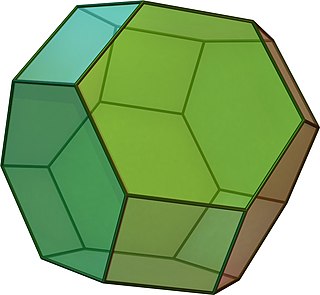
In geometry, the truncated octahedron is the Archimedean solid that arises from a regular octahedron by removing six pyramids, one at each of the octahedron's vertices. The truncated octahedron has 14 faces, 36 edges, and 24 vertices. Since each of its faces has point symmetry the truncated octahedron is a 6-zonohedron. It is also the Goldberg polyhedron GIV(1,1), containing square and hexagonal faces. Like the cube, it can tessellate 3-dimensional space, as a permutohedron.

In geometry, the triangular bipyramid is a type of hexahedron, being the first in the infinite set of face-transitive bipyramids. It is the dual of the triangular prism with 6 isosceles triangle faces.

The triaugmented triangular prism, in geometry, is a convex polyhedron with 14 equilateral triangles as its faces. It can be constructed from a triangular prism by attaching equilateral square pyramids to each of its three square faces. The same shape is also called the tetrakis triangular prism, tricapped trigonal prism, tetracaidecadeltahedron, or tetrakaidecadeltahedron; these last names mean a polyhedron with 14 triangular faces. It is an example of a deltahedron and of a Johnson solid.

In geometry, a square pyramid is a pyramid with a square base, having a total of five faces. If the apex of the pyramid is directly above the center of the square, it is a right square pyramid with four isosceles triangles; otherwise, it is an oblique square pyramid. When all of the pyramid's edges are equal in length, its triangles are all equilateral, and it is called an equilateral square pyramid.

In geometry, the square cupola, sometimes called lesser dome, is one of the Johnson solids. It can be obtained as a slice of the rhombicuboctahedron. As in all cupolae, the base polygon has twice as many edges and vertices as the top; in this case the base polygon is an octagon.

In geometry, the gyroelongated square cupola is one of the Johnson solids (J23). As the name suggests, it can be constructed by gyroelongating a square cupola (J4) by attaching an octagonal antiprism to its base. It can also be seen as a gyroelongated square bicupola (J45) with one square bicupola removed.

In geometry, the square gyrobicupola is one of the Johnson solids. Like the square orthobicupola, it can be obtained by joining two square cupolae along their bases. The difference is that in this solid, the two halves are rotated 45 degrees with respect to one another.

In geometry, the gyroelongated pentagonal birotunda is one of the Johnson solids. As the name suggests, it can be constructed by gyroelongating a pentagonal birotunda by inserting a decagonal antiprism between its two halves.
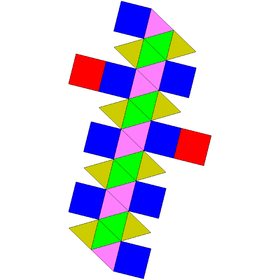
In geometry, the snub square antiprism is one of the Johnson solids . A Johnson solid is one of 92 strictly convex polyhedra that is composed of regular polygon faces but are not uniform polyhedra. They were named by Norman Johnson, who first listed these polyhedra in 1966.

In geometry, the gyroelongated pentagonal cupola is one of the Johnson solids (J24). As the name suggests, it can be constructed by gyroelongating a pentagonal cupola (J5) by attaching a decagonal antiprism to its base. It can also be seen as a gyroelongated pentagonal bicupola (J46) with one pentagonal cupola removed.

In geometry, the gyroelongated pentagonal bicupola is one of the Johnson solids. As the name suggests, it can be constructed by gyroelongating a pentagonal bicupola by inserting a decagonal antiprism between its congruent halves.

In geometry, the gyroelongated triangular cupola is one of the Johnson solids (J22). It can be constructed by attaching a hexagonal antiprism to the base of a triangular cupola (J3). This is called "gyroelongation", which means that an antiprism is joined to the base of a solid, or between the bases of more than one solid.

In geometry, the gyroelongated triangular bicupola is one of the Johnson solids. As the name suggests, it can be constructed by gyroelongating a triangular bicupola by inserting a hexagonal antiprism between its congruent halves.

In geometry, the gyroelongated pentagonal cupolarotunda is one of the Johnson solids. As the name suggests, it can be constructed by gyroelongating a pentagonal cupolarotunda by inserting a decagonal antiprism between its two halves.

In geometry, a bicupola is a solid formed by connecting two cupolae on their bases.

In geometry, the medial rhombic triacontahedron is a nonconvex isohedral polyhedron. It is a stellation of the rhombic triacontahedron, and can also be called small stellated triacontahedron. Its dual is the dodecadodecahedron.

In geometry, an octadecahedron is a polyhedron with 18 faces. No octadecahedron is regular; hence, the name does not commonly refer to one specific polyhedron.