1895 in science

Last updated

List of years in science (table)
+...

The year 1895 in science and technology involved some significant events, listed below.

Contents

Astronomy and space sciences

Biology

Chemistry

Climatology

Ecology

Mathematics

Medicine

Physics

Psychiatry

Technology

Other events

Awards

Births

Deaths

Related Research Articles

<span class="mw-page-title-main">Helium</span> Chemical element with atomic number 2 (He)

Helium is a chemical element; it has symbol He and atomic number 2. It is a colorless, odorless, non-toxic, inert, monatomic gas and the first in the noble gas group in the periodic table. Its boiling point is the lowest among all the elements, and it does not have a melting point at standard pressures. It is the second-lightest and second most abundant element in the observable universe, after hydrogen. It is present at about 24% of the total elemental mass, which is more than 12 times the mass of all the heavier elements combined. Its abundance is similar to this in both the Sun and Jupiter, because of the very high nuclear binding energy of helium-4, with respect to the next three elements after helium. This helium-4 binding energy also accounts for why it is a product of both nuclear fusion and radioactive decay. The most common isotope of helium in the universe is helium-4, the vast majority of which was formed during the Big Bang. Large amounts of new helium are created by nuclear fusion of hydrogen in stars.

<span class="mw-page-title-main">Soliton</span> Self-reinforcing single wave packet

In mathematics and physics, a soliton is a nonlinear, self-reinforcing, localized wave packet that is strongly stable, in that it preserves its shape while propagating freely, at constant velocity, and recovers it even after collisions with other such localized wave packets. Its remarkable stability can be traced to a balanced cancellation of nonlinear and dispersive effects in the medium. Solitons were subsequently found to provide stable solutions of a wide class of weakly nonlinear dispersive partial differential equations describing physical systems.

<span class="mw-page-title-main">Henri Becquerel</span> French physicist and engineer (1852–1908)

Antoine Henri Becquerel was a French engineer, physicist, Nobel laureate, and the first person to discover radioactivity. For work in this field he, along with Marie Skłodowska-Curie and Pierre Curie, received the 1903 Nobel Prize in Physics. The SI unit for radioactivity, the becquerel (Bq), is named after him.

<span class="mw-page-title-main">Wilhelm Röntgen</span> Discoverer of X-rays (1845–1923)

Wilhelm Conrad Röntgen was a German mechanical engineer and physicist, who, on 8 November 1895, produced and detected electromagnetic radiation in a wavelength range known as X-rays or Röntgen rays, an achievement that earned him the inaugural Nobel Prize in Physics in 1901. In honour of Röntgen's accomplishments, in 2004 the International Union of Pure and Applied Chemistry (IUPAC) named element 111, roentgenium, a radioactive element with multiple unstable isotopes, after him. The unit of measurement roentgen was also named after him.

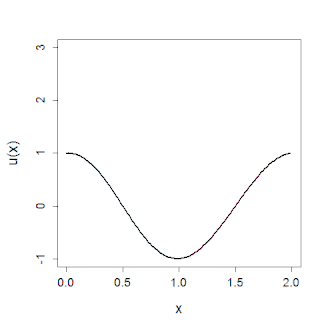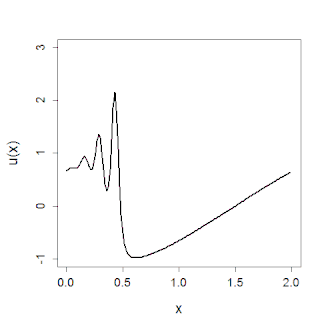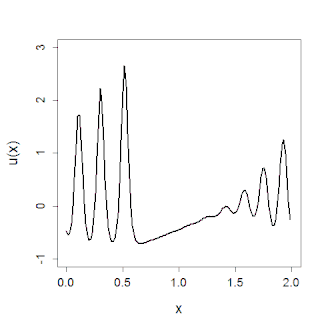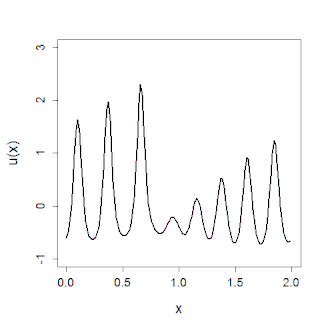
<span class="mw-page-title-main">Korteweg–De Vries equation</span> Mathematical model of waves on a shallow water surface

In mathematics, the Korteweg–De Vries (KdV) equation is a partial differential equation (PDE) which serves as a mathematical model of waves on shallow water surfaces. It is particularly notable as the prototypical example of an integrable PDE and exhibits many of the expected behaviors for an integrable PDE, such as a large number of explicit solutions, in particular soliton solutions, and an infinite number of conserved quantities, despite the nonlinearity which typically renders PDEs intractable. The KdV can be solved by the inverse scattering method (ISM). In fact, Gardner, Greene, Kruskal and Miura developed the classical inverse scattering method to solve the KdV equation.

The year 1888 in science and technology involved some significant events, listed below.

<span class="mw-page-title-main">Per Teodor Cleve</span> Swedish chemist who discovered holmium and thulium (1840–1905)

Per Teodor Cleve was a Swedish chemist, biologist, mineralogist and oceanographer. He is best known for his discovery of the chemical elements holmium and thulium.

The year 1937 in science and technology involved some significant events, listed below.

The year 1923 in science and technology involved some significant events, listed below.

The year 1845 in science and technology involved some significant events, listed below.

The year 1889 in science and technology involved some significant events, listed below.

The year 1868 in science and technology involved some significant events, listed below.

<span class="mw-page-title-main">Jean Baptiste Perrin</span> French physicist (1870–1942)

Jean Baptiste Perrin was a French physicist who, in his studies of the Brownian motion of minute particles suspended in liquids, verified Albert Einstein's explanation of this phenomenon and thereby confirmed the atomic nature of matter. For this achievement he was honoured with the Nobel Prize in Physics in 1926.

The year 1925 in science and technology involved some significant events, listed below.

<span class="mw-page-title-main">Cleveite</span> Radioactive variety of uraninite

Cleveite is an impure radioactive variety of uraninite containing uranium, found in Norway. It has the composition UO2 with about 10% of the uranium substituted by rare-earth elements. It was named after Swedish chemist Per Teodor Cleve.

<span class="mw-page-title-main">Emil Fischer</span> German chemist (1852–1919)

Hermann Emil Louis Fischer was a German chemist and 1902 recipient of the Nobel Prize in Chemistry. He discovered the Fischer esterification. He also developed the Fischer projection, a symbolic way of drawing asymmetric carbon atoms. He also hypothesized lock and key mechanism of enzyme action. He never used his first given name, and was known throughout his life simply as Emil Fischer.

<span class="mw-page-title-main">Diederik Korteweg</span> Dutch mathematician (1848–1941)

Diederik Johannes Korteweg was a Dutch mathematician. He is now best remembered for his work on the Korteweg–de Vries equation, together with Gustav de Vries.

<span class="mw-page-title-main">Gustav de Vries</span> Dutch mathematician (1866–1934)

Gustav de Vries was a Dutch mathematician, who is best remembered for his work on the Korteweg–de Vries equation with Diederik Korteweg. He was born on 22 January 1866 in Amsterdam, and studied at the University of Amsterdam with the distinguished physical chemist Johannes van der Waals and with Korteweg. While doing his doctoral research De Vries supported himself by teaching at the Royal Military Academy in Breda (1892-1893) and at the "cadettenschool" in Alkmaar (1893-1894). Under Korteweg's supervision De Vries completed his doctoral dissertation: Bijdrage tot de kennis der lange golven, Acad. proefschrift, Universiteit van Amsterdam, 1894, 95 pp, Loosjes, Haarlem. The following year Korteweg and De Vries published the research paper On the Change of Form of Long Waves advancing in a Rectangular Canal and on a New Type of Long Stationary Waves, Philosophical Magazine, 5th series, 39, 1895, pp. 422–443. In 1894 De Vries worked as a high school teacher at the "HBS en Handelsschool" in Haarlem, where he remained until his retirement in 1931. He died in Haarlem on 16 December 1934. The Korteweg-de Vries Institute for Mathematics is named after him.

Events in the year 1895 in Germany.

References

  1. ↑ "Gov. Morton Signs the Bill Providing for a Zoological Garden in This City". The Sun . April 27, 1895. p. 7. Retrieved January 20, 2023– via Newspapers.com.
  2. ↑ Bruce, David (1895). Preliminary Report on Tsetse Fly Disease or Nagana in Zululand. Durban, South Africa: Bennet & Davis. OCLC   14776707 – via Internet Archive.
  3. ↑ Duggan, A. J. (1977). "Bruce and the African Trypanosomes" . The American Journal of Tropical Medicine and Hygiene . 26 (5 Pt 2 Suppl): 1080–3. doi:10.4269/ajtmh.1977.26.1080. PMID   20787.
  4. ↑ Brandt, L W (1968). "Helium". In Hampel, Clifford A. (ed.). The Encyclopedia of the Chemical Elements . New York: Van Nostrand Reinhold. pp. 256–7. ISBN   0-442-15598-0 – via Open Library.
  5. ↑ Munday P (1999). "Hillebrand, William Francis (12 Dec. 1853–7 Feb. 1925)". In Garrary JA, Carnes MC (eds.). American National Biography . Vol. 10. Oxford University Press. p. 808 – via Internet Archive.
  6. ↑ Ramsay, William (1895). "On a Gas Showing the Spectrum of Helium, the Reputed Cause of D3, one of the lines in the Coronal Spectrum. Preliminary Note". Proceedings of the Royal Society of London. 58 (1): 65–67. doi: 10.1098/rspl.1895.0006 .
  7. ↑ Emsley, John (2001). "Helium". Nature's Building Blocks (2002 reprint ed.). Oxford University Press. p. 177. ISBN   0-19-850341-5 – via Open Library.
  8. ↑ Langlet, N. A. (1895). "Über das Atomgewicht des Heliums" [About the atomic weight of helium]. Zeitschrift für Anorganische Chemie (in German). 10 (1): 289–292. doi: 10.1002/zaac.18950100130 – via Zenodo.
  9. ↑ Fischer, Emil; Speier, Arthur (December 1895). "Darstellung der Ester" [Representation of the Esters]. Chemische Berichte . 28 (3): 3252–3258. doi:10.1002/cber.189502803176.
  10. ↑ "On the Influence of Carbonic Acid in the Air Upon The Temperature of the Ground". The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science . April 1896.
  11. ↑ "The Endangered Species Act – History Of Species Protection". Science Encyclopedia. Library Index. Archived from the original on 2013-07-01. Retrieved 2012-11-18.
  12. ↑ Korteweg, D. J.; de Vries, G. (1895). "On the Change of Form of Long Waves Advancing in a Rectangular Canal, and on a New Type of Long Stationary Waves". The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science . 39 (240): 422–443. doi:10.1080/14786449508620739.
  13. ↑ Cantor, Georg (1895). "Beiträge zur Begründung der transfiniten Mengenlehre (1)". Mathematische Annalen . 46 (4): 481–512. doi:10.1007/bf02124929. S2CID   177801164. Archived from the original on 2014-04-23.
  14. ↑ Poincaré, Henri (1895). "Analysis situs". Journal de l'École Polytechnique. (2). 1. Paris: 1–123.
  15. ↑ "Report 12 of the Council on Scientific Affairs (A-97)". American Medical Association. 1997. Archived from the original on 2009-06-14.
  16. ↑ "Popov's Contribution to the Development of Wireless Communication, 1895". IEEE Global History Network. Milestones. IEEE . Retrieved 2013-02-23.
  17. ↑ Röntgen, W. (December 1895). "Eine neue Art von Strahlen". Sityzungs-Berichteder Physikalisch-medicinisch Gesellschaft zu Würzburg (9).
  18. ↑ Chardère, B.; Borgé, G.; Borgé, M. (1985). Les Lumière (in French). Paris: Bibliothèque des Arts. p. 71. ISBN   2-85047-068-6.
  19. ↑ "New "L" Road Opens". Chicago Daily Tribune . 1895-05-07. p. 12.
  20. ↑ "Cermak Road Bridge District" (PDF). City of Chicago. 2003. p. 17. Archived from the original (PDF) on January 31, 2012. Retrieved 2012-02-09.
  21. ↑ Tschöke, Helmut; Mollenhauer, Klaus; Maier, Rudolf, eds. (2018). Handbuch Dieselmotoren (8th ed.). Wiesbaden: Springer. p. 6. ISBN   978-3-658-07696-2.
  22. ↑ Curry, Roger (2021). Engines of Change. p. 217.
  23. ↑ "Electrical Bicycle". Google Patents. 1895. Retrieved 2012-05-25.
  24. ↑ Morchin, William C.; Oman, Henry (2006). Electric Bicycles . Hoboken: Wiley. p.  3. ISBN   978-0-471-67419-1.
  25. ↑ File:The Engineering and Mining Journal 1897-12-18- Vol 64 Iss 25 (IA sim engineering-and-mining-journal 1897-12-18 64 25).pdf
  26. ↑ "Copley Medal | British scientific award". Encyclopedia Britannica. Retrieved 23 July 2020.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.