In physics and general relativity, gravitational redshift is the phenomenon that electromagnetic waves or photons travelling out of a gravitational well lose energy. This loss of energy corresponds to a decrease in the wave frequency and increase in the wavelength, known more generally as a redshift. The opposite effect, in which photons gain energy when travelling into a gravitational well, is known as a gravitational blueshift. The effect was first described by Einstein in 1907, eight years before his publication of the full theory of relativity.

Oscillation is the repetitive or periodic variation, typically in time, of some measure about a central value or between two or more different states. Familiar examples of oscillation include a swinging pendulum and alternating current. Oscillations can be used in physics to approximate complex interactions, such as those between atoms.

In particle physics, bremsstrahlung is electromagnetic radiation produced by the deceleration of a charged particle when deflected by another charged particle, typically an electron by an atomic nucleus. The moving particle loses kinetic energy, which is converted into radiation, thus satisfying the law of conservation of energy. The term is also used to refer to the process of producing the radiation. Bremsstrahlung has a continuous spectrum, which becomes more intense and whose peak intensity shifts toward higher frequencies as the change of the energy of the decelerated particles increases.

In optics, a Fabry–Pérot interferometer (FPI) or etalon is an optical cavity made from two parallel reflecting surfaces. Optical waves can pass through the optical cavity only when they are in resonance with it. It is named after Charles Fabry and Alfred Perot, who developed the instrument in 1899. Etalon is from the French étalon, meaning "measuring gauge" or "standard".

Asteroseismology is the study of oscillations in stars. Stars have many resonant modes and frequencies, and the path of sound waves passing through a star depends on the speed of sound, which in turn depends on local temperature and chemical composition. Because the resulting oscillation modes are sensitive to different parts of the star, they inform astronomers about the internal structure of the star, which is otherwise not directly possible from overall properties like brightness and surface temperature.
Helioseismology, a term coined by Douglas Gough, is the study of the structure and dynamics of the Sun through its oscillations. These are principally caused by sound waves that are continuously driven and damped by convection near the Sun's surface. It is similar to geoseismology, or asteroseismology, which are respectively the studies of the Earth or stars through their oscillations. While the Sun's oscillations were first detected in the early 1960s, it was only in the mid-1970s that it was realized that the oscillations propagated throughout the Sun and could allow scientists to study the Sun's deep interior. The modern field is separated into global helioseismology, which studies the Sun's resonant modes directly, and local helioseismology, which studies the propagation of the component waves near the Sun's surface.
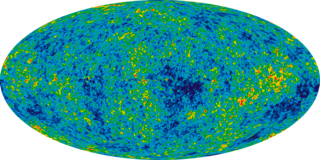
Primordial fluctuations are density variations in the early universe which are considered the seeds of all structure in the universe. Currently, the most widely accepted explanation for their origin is in the context of cosmic inflation. According to the inflationary paradigm, the exponential growth of the scale factor during inflation caused quantum fluctuations of the inflaton field to be stretched to macroscopic scales, and, upon leaving the horizon, to "freeze in". At the later stages of radiation- and matter-domination, these fluctuations re-entered the horizon, and thus set the initial conditions for structure formation.

Neutrino oscillation is a quantum mechanical phenomenon in which a neutrino created with a specific lepton family number can later be measured to have a different lepton family number. The probability of measuring a particular flavor for a neutrino varies between three known states, as it propagates through space.

The cosmic neutrino background is the universe's background particle radiation composed of neutrinos. They are sometimes known as relic neutrinos.

A satellite galaxy is a smaller companion galaxy that travels on bound orbits within the gravitational potential of a more massive and luminous host galaxy. Satellite galaxies and their constituents are bound to their host galaxy, in the same way that planets within our own solar system are gravitationally bound to the Sun. While most satellite galaxies are dwarf galaxies, satellite galaxies of large galaxy clusters can be much more massive. The Milky Way is orbited by about fifty satellite galaxies, the largest of which is the Large Magellanic Cloud.
In theoretical physics, massive gravity is a theory of gravity that modifies general relativity by endowing the graviton with a nonzero mass. In the classical theory, this means that gravitational waves obey a massive wave equation and hence travel at speeds below the speed of light.
In theoretical physics, a scalar–tensor theory is a field theory that includes both a scalar field and a tensor field to represent a certain interaction. For example, the Brans–Dicke theory of gravitation uses both a scalar field and a tensor field to mediate the gravitational interaction.
In particle physics, the Pontecorvo–Maki–Nakagawa–Sakata matrix, Maki–Nakagawa–Sakata matrix, lepton mixing matrix, or neutrino mixing matrix is a unitary mixing matrix which contains information on the mismatch of quantum states of neutrinos when they propagate freely and when they take part in weak interactions. It is a model of neutrino oscillation. This matrix was introduced in 1962 by Ziro Maki, Masami Nakagawa, and Shoichi Sakata, to explain the neutrino oscillations predicted by Bruno Pontecorvo.
f(R) is a type of modified gravity theory which generalizes Einstein's general relativity. f(R) gravity is actually a family of theories, each one defined by a different function, f, of the Ricci scalar, R. The simplest case is just the function being equal to the scalar; this is general relativity. As a consequence of introducing an arbitrary function, there may be freedom to explain the accelerated expansion and structure formation of the Universe without adding unknown forms of dark energy or dark matter. Some functional forms may be inspired by corrections arising from a quantum theory of gravity. f(R) gravity was first proposed in 1970 by Hans Adolph Buchdahl. It has become an active field of research following work by Starobinsky on cosmic inflation. A wide range of phenomena can be produced from this theory by adopting different functions; however, many functional forms can now be ruled out on observational grounds, or because of pathological theoretical problems.
In the context of the physical and mathematical theory of percolation, a percolation transition is characterized by a set of universal critical exponents, which describe the fractal properties of the percolating medium at large scales and sufficiently close to the transition. The exponents are universal in the sense that they only depend on the type of percolation model and on the space dimension. They are expected to not depend on microscopic details such as the lattice structure, or whether site or bond percolation is considered. This article deals with the critical exponents of random percolation.
Lorentz-violating neutrino oscillation refers to the quantum phenomenon of neutrino oscillations described in a framework that allows the breakdown of Lorentz invariance. Today, neutrino oscillation or change of one type of neutrino into another is an experimentally verified fact; however, the details of the underlying theory responsible for these processes remain an open issue and an active field of study. The conventional model of neutrino oscillations assumes that neutrinos are massive, which provides a successful description of a wide variety of experiments; however, there are a few oscillation signals that cannot be accommodated within this model, which motivates the study of other descriptions. In a theory with Lorentz violation, neutrinos can oscillate with and without masses and many other novel effects described below appear. The generalization of the theory by incorporating Lorentz violation has shown to provide alternative scenarios to explain all the established experimental data through the construction of global models.
Laser linewidth is the spectral linewidth of a laser beam.

Kapitza's pendulum or Kapitza pendulum is a rigid pendulum in which the pivot point vibrates in a vertical direction, up and down. It is named after Russian Nobel laureate physicist Pyotr Kapitza, who in 1951 developed a theory which successfully explains some of its unusual properties. The unique feature of the Kapitza pendulum is that the vibrating suspension can cause it to balance stably in an inverted position, with the bob above the suspension point. In the usual pendulum with a fixed suspension, the only stable equilibrium position is with the bob hanging below the suspension point; the inverted position is a point of unstable equilibrium, and the smallest perturbation moves the pendulum out of equilibrium. In nonlinear control theory the Kapitza pendulum is used as an example of a parametric oscillator that demonstrates the concept of "dynamic stabilization".
In quantum physics, light is in a squeezed state if its electric field strength Ԑ for some phases has a quantum uncertainty smaller than that of a coherent state. The term squeezing thus refers to a reduced quantum uncertainty. To obey Heisenberg's uncertainty relation, a squeezed state must also have phases at which the electric field uncertainty is anti-squeezed, i.e. larger than that of a coherent state. Since 2019, the gravitational-wave observatories LIGO and Virgo employ squeezed laser light, which has significantly increased the rate of observed gravitational-wave events.

FG Virginis is a well-studied variable star in the equatorial constellation of Virgo. It is a dim star, near the lower limit of visibility to the naked eye, with an apparent visual magnitude that ranges from 6.53 down to 6.58. The star is located at a distance of 273.5 light years from the Sun based on parallax measurements, and is drifting further away with a radial velocity of +16 km/s. Because of its position near the ecliptic, it is subject to lunar occultations.



















