Lunar distance (navigation)

Last updated
Finding Greenwich time while at sea using a lunar distance. The lunar distance is the angle between the Moon and a star (or the Sun). In the above illustration the star Regulus is used. The altitudes of the two bodies are used to make corrections and determine the time. Lunars-star-map.jpg
Finding Greenwich time while at sea using a lunar distance. The lunar distance is the angle between the Moon and a star (or the Sun). In the above illustration the star Regulus is used. The altitudes of the two bodies are used to make corrections and determine the time.

In celestial navigation, lunar distance, also called a lunar, is the angular distance between the Moon and another celestial body. The lunar distances method uses this angle and a nautical almanac to calculate Greenwich time if so desired, or by extension any other time. That calculated time can be used in solving a spherical triangle. The theory was first published by Johannes Werner in 1524, before the necessary almanacs had been published. A fuller method was published in 1763 and used until about 1850 when it was superseded by the marine chronometer. A similar method uses the positions of the Galilean moons of Jupiter.

Contents

Purpose

In celestial navigation, knowledge of the time at Greenwich (or another known place) and the measured positions of one or more celestial objects allows the navigator to calculate latitude and longitude. [1] Reliable marine chronometers were unavailable until the late 18th century and not affordable until the 19th century. [2] [3] [4] After the method was first published in 1763 by British Astronomer Royal Nevil Maskelyne, based on pioneering work by Tobias Mayer, for about a hundred years (until about 1850) [5] mariners lacking a chronometer used the method of lunar distances to determine Greenwich time as a key step in determining longitude. Conversely, a mariner with a chronometer could check its accuracy using a lunar determination of Greenwich time. [2] The method saw usage all the way up to the beginning of the 20th century on smaller vessels that could not afford a chronometer or had to rely on this technique for correction of the chronometer. [6]

Method

Summary

The method relies on the relatively quick movement of the moon across the background sky, completing a circuit of 360 degrees in 27.3 days (the sidereal month), or 13.2 degrees per day. In one hour it will move approximately half a degree, [1] roughly its own angular diameter, with respect to the background stars and the Sun.

Using a sextant, the navigator precisely measures the angle between the moon and another body. [1] That could be the Sun or one of a selected group of bright stars lying close to the Moon's path, near the ecliptic. At that moment, anyone on the surface of the earth who can see the same two bodies will, after correcting for parallax, observe the same angle. The navigator then consults a prepared table of lunar distances and the times at which they will occur. [1] [7] By comparing the corrected lunar distance with the tabulated values, the navigator finds the Greenwich time for that observation. Knowing Greenwich time and local time, the navigator can work out longitude. [1]

Local time can be determined from a sextant observation of the altitude of the Sun or a star. [8] [9] Then the longitude (relative to Greenwich) is readily calculated from the difference between local time and Greenwich Time, at 15 degrees per hour of difference.

In practice

Having measured the lunar distance and the heights of the two bodies, the navigator can find Greenwich time in three steps:

  1. Preliminaries: Almanac tables predict lunar distances between the centre of the Moon and the other body (published between 1767 and 1906 in Britain). [10] [11] However, the observer cannot accurately find the centre of the Moon (or Sun, which was the most frequently used second object). Instead, lunar distances are always measured to the sharply lit, outer edge (the limb, not terminator) of the Moon (or of the Sun). The first correction to the lunar distance is the distance between the limb of the Moon and its center. Since the Moon's apparent size varies with its varying distance from the Earth, almanacs give the Moon's and Sun's semidiameter for each day. [12] Additionally the observed altitudes are cleared of semidiameter.
  2. Clearing: The lunar distance is corrected for the effects of parallax and atmospheric refraction on the observation. The almanac gives lunar distances as they would appear if the observer were at the center of a transparent Earth. Because the Moon is so much closer to the Earth than the stars are, the position of the observer on the surface of the Earth shifts the relative position of the Moon by up to an entire degree. [13] [14] The clearing correction for parallax and refraction is a trigonometric function of the observed lunar distance and the altitudes of the two bodies. [15] Navigators used collections of mathematical tables to work these calculations by any of dozens of distinct clearing methods. For practical applications today the tables by Bruce Stark [16] may be used for clearing the lunar distance. They are constructed such that only additions and subtractions of tabulated numbers are required instead of trigonometric evaluations.
  3. Finding the time: The navigator, having cleared the lunar distance, now consults a prepared table of lunar distances and the times at which they will occur in order to determine the Greenwich time of the observation. [1] [7] Predicting the position of the moon years in advance requires solving the three-body problem, since the earth, moon and sun were all involved. Euler developed the numerical method they used, called Euler's method, and received a grant from the Board of Longitude to carry out the computations.

Having found the (absolute) Greenwich time, the navigator either compares it with the observed local apparent time (a separate observation) to find his longitude, or compares it with the Greenwich time on a chronometer (if available) if one wants to check the chronometer. [1]

Errors

Almanac error

By 1810, the errors in the almanac predictions had been reduced to about one-quarter of a minute of arc. By about 1860 (after lunar distance observations had mostly faded into history), the almanac errors were finally reduced to less than the error margin of a sextant in ideal conditions (one-tenth of a minute of arc).

Lunar distance observation

Later sextants (after c. 1800) could indicate angle to 0.1 arc-minutes, after the use of the vernier was popularized by its description in English in the book Navigatio Britannica published in 1750 by John Barrow, the mathematician and historian. In practice at sea, actual errors were somewhat larger. If the sky is cloudy or the Moon is new (hidden close to the glare of the Sun), lunar distance observations could not be performed.

Total error

A lunar distance changes with time at a rate of roughly half a degree, or 30 arc-minutes, in an hour. [1] The two sources of error, combined, typically amount to about one-half arc-minute in Lunar distance, equivalent to one minute in Greenwich time, which corresponds to an error of as much as one-quarter of a degree of longitude, or about 15 nautical miles (28 km) at the equator.

In literature

Captain Joshua Slocum, in making the first solo circumnavigation of the Earth in 1895–1898, somewhat anachronistically used the lunar method along with dead reckoning in his navigation. He comments in Sailing Alone Around the World on a sight taken in the South Pacific. After correcting an error he found in his log tables, the result was surprisingly accurate: [17]

I found from the result of three observations, after long wrestling with lunar tables, that her longitude agreed within five miles of that by dead-reckoning. This was wonderful; both, however, might be in error, but somehow I felt confident that both were nearly true, and that in a few hours more I should see land; and so it happened, for then I made out the island of Nukahiva, the southernmost of the Marquesas group, clear-cut and lofty. The verified longitude when abreast was somewhere between the two reckonings; this was extraordinary. All navigators will tell you that from one day to another a ship may lose or gain more than five miles in her sailing-account, and again, in the matter of lunars, even expert lunarians are considered as doing clever work when they average within eight miles of the truth...

The result of these observations naturally tickled my vanity, for I knew it was something to stand on a great ship’s deck and with two assistants take lunar observations approximately near the truth. As one of the poorest of American sailors, I was proud of the little achievement alone on the sloop, even by chance though it may have been...

The work of the lunarian, though seldom practised in these days of chronometers, is beautifully edifying, and there is nothing in the realm of navigation that lifts one’s heart up more in adoration.

In his 1777 book, "A Voyage around the World", naturalist Georg Forster described his impressions of navigation with captain James Cook on board the ship HMS Resolution in the South Pacific. Cook had two of the new chronometers on board, one made by Larcum Kendall the other by John Arnold, following the lead of the famous John Harrison clocks. On March 12, 1774, approaching Easter Island, Forster found praiseworthy the method of lunar distances as the best and most precise method to determine longitude, as compared to clocks which may fail due to mechanical problems.

See also

Related Research Articles

<span class="mw-page-title-main">Longitude</span> Geographic coordinate that specifies the east-west position of a point on the Earths surface

Longitude is a geographic coordinate that specifies the east–west position of a point on the surface of the Earth, or another celestial body. It is an angular measurement, usually expressed in degrees and denoted by the Greek letter lambda (λ). Meridians are imaginary semicircular lines running from pole to pole that connect points with the same longitude. The prime meridian defines 0° longitude; by convention the International Reference Meridian for the Earth passes near the Royal Observatory in Greenwich, south-east London on the island of Great Britain. Positive longitudes are east of the prime meridian, and negative ones are west.

<span class="mw-page-title-main">Navigation</span> Process of monitoring and controlling the movement of a craft or vehicle from one place to another

Navigation is a field of study that focuses on the process of monitoring and controlling the movement of a craft or vehicle from one place to another. The field of navigation includes four general categories: land navigation, marine navigation, aeronautic navigation, and space navigation.

<span class="mw-page-title-main">Sextant</span> Tool for angle measurement

A sextant is a doubly reflecting navigation instrument that measures the angular distance between two visible objects. The primary use of a sextant is to measure the angle between an astronomical object and the horizon for the purposes of celestial navigation.

<span class="mw-page-title-main">Longitude rewards</span> 1714 British prize for ships chronometers

The longitude rewards were the system of inducement prizes offered by the British government for a simple and practical method for the precise determination of a ship's longitude at sea. The prizes, established through an Act of Parliament in 1714, were administered by the Board of Longitude.

<span class="mw-page-title-main">Board of Longitude</span> British government department tasked with improving accurate marine chronometry

The Commissioners for the Discovery of the Longitude at Sea, or more popularly Board of Longitude, was a British government body formed in 1714 to administer a scheme of prizes intended to encourage innovators to solve the problem of finding longitude at sea.

<span class="mw-page-title-main">Celestial navigation</span> Navigation using astronomical objects to determine position

Celestial navigation, also known as astronavigation, is the practice of position fixing using stars and other celestial bodies that enables a navigator to accurately determine their actual current physical position in space or on the surface of the Earth without relying solely on estimated positional calculations, commonly known as dead reckoning. Celestial navigation is performed without using satellite navigation or other similar modern electronic or digital positioning means.

<span class="mw-page-title-main">Nautical almanac</span>

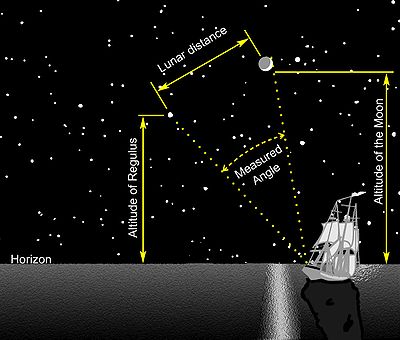
A nautical almanac is a publication describing the positions of a selection of celestial bodies for the purpose of enabling navigators to use celestial navigation to determine the position of their ship while at sea. The Almanac specifies for each whole hour of the year the position on the Earth's surface at which the Sun, Moon, planets, and First Point of Aries is directly overhead. The positions of 57 selected stars are specified relative to the First Point of Aries.

In astronomical navigation, the intercept method, also known as Marcq St. Hilaire method, is a method of calculating an observer's position on Earth (geopositioning). It was originally called the azimuth intercept method because the process involves drawing a line which intercepts the azimuth line. This name was shortened to intercept method and the intercept distance was shortened to 'intercept'.

<span class="mw-page-title-main">Longitude by chronometer</span>

Longitude by chronometer is a method, in navigation, of determining longitude using a marine chronometer, which was developed by John Harrison during the first half of the eighteenth century. It is an astronomical method of calculating the longitude at which a position line, drawn from a sight by sextant of any celestial body, crosses the observer's assumed latitude. In order to calculate the position line, the time of the sight must be known so that the celestial position i.e. the Greenwich Hour Angle and Declination, of the observed celestial body is known. All that can be derived from a single sight is a single position line, which can be achieved at any time during daylight when both the sea horizon and the sun are visible. To achieve a fix, more than one celestial body and the sea horizon must be visible. This is usually only possible at dawn and dusk.

Ex-meridian is a celestial navigation method of calculating an observer's position on Earth. The method gives the observer a position line on which the observer is situated. It is usually used when the Sun is obscured at noon, and as a result, a meridian altitude is not possible. The navigator measures the altitude of the Sun as close to noon as possible and then calculates where the position line lies.

Meridian altitude is a method of celestial navigation to calculate an observer's latitude. It notes the altitude angle of an astronomical object above the horizon at culmination.

The navigational triangle or PZX triangle is a spherical triangle used in astronavigation to determine the observer's position on the globe. It is composed of three reference points on the celestial sphere:

<span class="mw-page-title-main">Marine chronometer</span> Clock used on ships to aid in navigation

A marine chronometer is a precision timepiece that is carried on a ship and employed in the determination of the ship's position by celestial navigation. It is used to determine longitude by comparing Greenwich Mean Time (GMT), and the time at the current location found from observations of celestial bodies. When first developed in the 18th century, it was a major technical achievement, as accurate knowledge of the time over a long sea voyage was vital for effective navigation, lacking electronic or communications aids. The first true chronometer was the life work of one man, John Harrison, spanning 31 years of persistent experimentation and testing that revolutionized naval navigation.

<span class="mw-page-title-main">History of longitude</span> Record of humanitys attempts to find east-west position on Earth

The history of longitude describes the centuries-long effort by astronomers, cartographers and navigators to discover a means of determining the longitude of any given place on Earth. The measurement of longitude is important to both cartography and navigation. In particular, for safe ocean navigation, knowledge of both latitude and longitude is required, however latitude can be determined with good accuracy with local astronomical observations.

Nautical time is a maritime time standard established in the 1920s to allow ships on high seas to coordinate their local time with other ships, consistent with a long nautical tradition of accurate celestial navigation. Nautical time divides the globe into 24 nautical time zones with hourly clock offsets, spaced at 15 degrees by longitudinal coordinate, with no political deviation.

<i>The Nautical Almanac</i>

The Nautical Almanac has been the familiar name for a series of official British almanacs published under various titles since the first issue of The Nautical Almanac and Astronomical Ephemeris, for 1767: this was the first nautical almanac to contain data dedicated to the convenient determination of longitude at sea. It was originally published from the Royal Greenwich Observatory in England. A detailed account of how the publication was produced in its earliest years has been published by the National Maritime Museum.

<span class="mw-page-title-main">Navigational algorithms</span>

The navigational algorithms are the quintessence of the executable software on portable calculators or Smartphone as an aid to the art of navigation, this attempt article describe both algorithms and software for "PC-Smartphone" implementing different calculation procedures for navigation . The calculation power obtained by the languages: Basic, "C", Java, etc. .., from portable calculators or Smartphones, has made it possible to develop programs that allow calculating the position without the need for tables, in fact they have some basic tables with the correction factors for each year and calculate the values "on the fly" at runtime.

<i>Longitude</i> (book) 1995 popular science book

Longitude: The True Story of a Lone Genius Who Solved the Greatest Scientific Problem of His Time is a 1995 best-selling book by Dava Sobel about John Harrison, an 18th-century clockmaker who created the first clock (chronometer) sufficiently accurate to be used to determine longitude at sea—an important development in navigation. The book was made into a television series entitled Longitude. In 1998, The Illustrated Longitude was published, supplementing the earlier text with 180 images of characters, events, instruments, maps and publications.

<span class="mw-page-title-main">Josef de Mendoza y Ríos</span> 18th and 19th-century Spanish astronomer and mathematician

Josefde Mendoza y Ríos (1761–1816) was a Spanish astronomer and mathematician of the 18th century, famous for his work on navigation. The first work of Mendoza y Ríos was published in 1787: his treatise about the science and technique of navigation in two tomes. He also published several tables for facilitating the calculations of nautical astronomy and useful in navigation to calculate the latitude of a ship at sea from two altitudes of the sun, and the longitude from the distances of the moon from a celestial body.

Janet Taylor was an English astronomer, navigation expert, mathematician, meteorologist, and founder of the George Taylor Nautical Academy. She was the author of various astronomy and navigation works, and owner of a navigational instrument production and repair warehouse.

References

  1. 1 2 3 4 5 6 7 8 Norie, J. W. (1828). New and Complete Epitome of Practical Navigation. London. p. 222. Archived from the original on 2007-09-27. Retrieved 2007-08-02.
  2. 1 2 Norie, J. W. (1828). New and Complete Epitome of Practical Navigation. London. p. 221. Archived from the original on 2007-09-27. Retrieved 2007-08-02.
  3. ↑ Taylor, Janet (1851). An Epitome of Navigation and Nautical Astronomy (Ninth ed.). Taylor. p. 295f. Retrieved 2007-08-02. Nautical Almanac 1849-1851.
  4. ↑ Britten, Frederick James (1894). Former Clock & Watchmakers and Their Work. New York: Spon & Chamberlain. p.  230 . Retrieved 2007-08-08. Chronometers were not regularly supplied to the Royal Navy until about 1825
  5. ↑ Lecky, Squire, Wrinkles in Practical Navigation
  6. ↑ Bowditch, Nathaniel (2002). "Chapter 1"  . The American Practical Navigator  . United States: National Imagery and Mapping Agency. p.  7  – via Wikisource.
  7. 1 2 Royal Greenwich Observatory. "DISTANCES of Moon's Center from Sun, and from Stars EAST of her". In Garnet (ed.). The Nautical Almanac and Astronomical Ephemeris for the year 1804 (Second American Impression ed.). New Jersey: Blauvelt. p. 92. Archived from the original on 2007-09-27. Retrieved 2007-08-02.;
    Wepster, Steven. "Precomputed Lunar Distances". Archived from the original on 2007-12-15. Retrieved 2007-08-02.
  8. ↑ Norie, J. W. (1828). New and Complete Epitome of Practical Navigation. London. p. 226. Archived from the original on 2007-09-27. Retrieved 2007-08-02.
  9. ↑ Norie, J. W. (1828). New and Complete Epitome of Practical Navigation. London. p. 230. Archived from the original on 2007-09-27. Retrieved 2007-08-02.
  10. ↑ The Nautical Almanac and Astronomical Ephemeris, for the year 1767, London: W. Richardson and S. Clark, 1766
  11. ↑ The Nautical Almanac Abridged for the Use of Seamen, 1924
  12. ↑ Dunlop, G.D.; Shufeldt, H.H. (1972). Dutton's navigation and Piloting. Annapolis, Maryland, USA: Naval Institute Press. p. 409. The authors show an example of correcting for lunar semidiameter.
  13. ↑ Duffett-Smith, Peter (1988). Practical Astronomy with your Calculator, third edition. Cambridge University Press. p. 66. ISBN   9780521356992.
  14. ↑ Montenbruck and Pfleger (1994). Astronomy on the Personal Computer, second edition. Springer. pp. 45–46. ISBN   9783540672210.
  15. ↑ Schlyter, Paul. "The Moon's topocentric position".
  16. ↑ Stark, Bruce (2010). Stark Tables for Clearing the Lunar Distance, third edition. Starpath Publications. ISBN   9780914025214.
  17. ↑ Captain Joshua Slocum, Sailing Alone Around the World, Chapter 11, 1900
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.