Gauss's law for magnetism

Last updated October 18, 2023

In physics, Gauss's law for magnetism is one of the four Maxwell's equations that underlie classical electrodynamics. It states that the magnetic field $B$ has divergence equal to zero,^[1] in other words, that it is a solenoidal vector field. It is equivalent to the statement that magnetic monopoles do not exist.^[2] Rather than "magnetic charges", the basic entity for magnetism is the magnetic dipole. (If monopoles were ever found, the law would have to be modified, as elaborated below.)

Differential form

The differential form for Gauss's law for magnetism is:

$\nabla \cdot \mathbf {B} =0$

where $\nabla \cdot$ denotes divergence, and $B$ is the magnetic field.

Integral form

Definition of a closed surface.
Left: Some examples of closed surfaces include the surface of a sphere, surface of a torus, and surface of a cube. The magnetic flux through any of these surfaces is zero.
Right: Some examples of non-closed surfaces include the disk surface, square surface, or hemisphere surface. They all have boundaries (red lines) and they do not fully enclose a 3D volume. The magnetic flux through these surfaces is not necessarily zero. SurfacesWithAndWithoutBoundary.svg — Definition of a closed surface.
**Left:** Some examples of closed surfaces include the surface of a sphere, surface of a torus, and surface of a cube. The magnetic flux through any of these surfaces is zero.
**Right:** Some examples of non-closed surfaces include the disk surface, square surface, or hemisphere surface. They all have boundaries (red lines) and they do not fully enclose a 3D volume. The magnetic flux through these surfaces is *not necessarily zero*.

The integral form of Gauss's law for magnetism states:

$\scriptstyle S$ $\mathbf {B} \cdot \mathrm {d} \mathbf {S} =0$

where $S$ is any closed surface (see image right), and $d S$ is a vector, whose magnitude is the area of an infinitesimal piece of the surface $S$ , and whose direction is the outward-pointing surface normal (see surface integral for more details).

The left-hand side of this equation is called the net flux of the magnetic field out of the surface, and Gauss's law for magnetism states that it is always zero.

The integral and differential forms of Gauss's law for magnetism are mathematically equivalent, due to the divergence theorem. That said, one or the other might be more convenient to use in a particular computation.

The law in this form states that for each volume element in space, there are exactly the same number of "magnetic field lines" entering and exiting the volume. No total "magnetic charge" can build up in any point in space. For example, the south pole of the magnet is exactly as strong as the north pole, and free-floating south poles without accompanying north poles (magnetic monopoles) are not allowed. In contrast, this is not true for other fields such as electric fields or gravitational fields, where total electric charge or mass can build up in a volume of space.

Vector potential

Due to the Helmholtz decomposition theorem, Gauss's law for magnetism is equivalent to the following statement:^[4]^[5]

There exists a vector field

A

such that

\mathbf {B} =\nabla \times \mathbf {A} .

The vector field $A$ is called the magnetic vector potential.

Note that there is more than one possible $A$ which satisfies this equation for a given $B$ field. In fact, there are infinitely many: any field of the form $\nabla ϕ$ can be added onto $A$ to get an alternative choice for $A$ , by the identity (see Vector calculus identities):

\nabla \times \mathbf {A} =\nabla \times (\mathbf {A} +\nabla \phi )

since the curl of a gradient is the zero vector field:

\nabla \times \nabla \phi ={\boldsymbol {0}}

This arbitrariness in $A$ is called gauge freedom.

Field lines

The magnetic field $B$ can be depicted via field lines (also called flux lines) – that is, a set of curves whose direction corresponds to the direction of $B$ , and whose areal density is proportional to the magnitude of $B$ . Gauss's law for magnetism is equivalent to the statement that the field lines have neither a beginning nor an end: Each one either forms a closed loop, winds around forever without ever quite joining back up to itself exactly, or extends to infinity.

Incorporating magnetic monopoles

If magnetic monopoles were to be discovered, then Gauss's law for magnetism would state the divergence of $B$ would be proportional to the magnetic charge density $ρ m$ , analogous to Gauss's law for electric field. For zero net magnetic charge density ( $ρ m = 0$ ), the original form of Gauss's magnetism law is the result.

The modified formula for use with the SI is not standard and depends on the choice of defining equation for the magnetic charge and current; in one variation, magnetic charge has units of webers, in another it has units of ampere-meters.

System	Equation
SI (weber convention)^[6]	$\nabla \cdot \mathbf {B} =\rho _{\mathrm {m} }$
SI (ampere-meter convention)^[7]	$\nabla \cdot \mathbf {B} =\mu _{0}\rho _{\mathrm {m} }$
CGS-Gaussian ^[8]	$\nabla \cdot \mathbf {B} =4\pi \rho _{\mathrm {m} }$

where $μ 0$ is the vacuum permeability.

So far, examples of magnetic monopoles are disputed in extensive search,^[9] although certain papers report examples matching that behavior. ^[10]

History

This idea of the nonexistence of the magnetic monopoles originated in 1269 by Petrus Peregrinus de Maricourt. His work heavily influenced William Gilbert, whose 1600 work De Magnete spread the idea further. In the early 1800s Michael Faraday reintroduced this law, and it subsequently made its way into James Clerk Maxwell's electromagnetic field equations.

Numerical computation

In numerical computation, the numerical solution may not satisfy Gauss's law for magnetism due to the discretization errors of the numerical methods. However, in many cases, e.g., for magnetohydrodynamics, it is important to preserve Gauss's law for magnetism precisely (up to the machine precision). Violation of Gauss's law for magnetism on the discrete level will introduce a strong non-physical force. In view of energy conservation, violation of this condition leads to a non-conservative energy integral, and the error is proportional to the divergence of the magnetic field.^[11]

There are various ways to preserve Gauss's law for magnetism in numerical methods, including the divergence-cleaning techniques,^[12] the constrained transport method,^[13] potential-based formulations^[14] and de Rham complex based finite element methods^[15]^[16] where stable and structure-preserving algorithms are constructed on unstructured meshes with finite element differential forms.

Related Research Articles

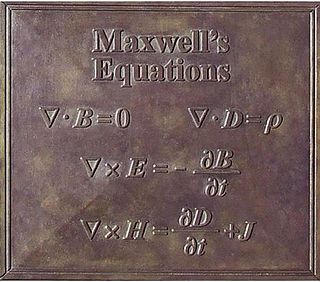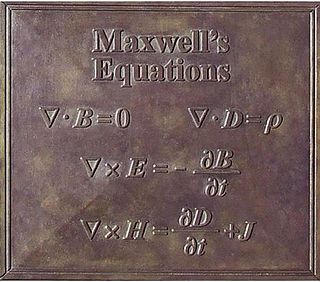
Maxwell's equations, or Maxwell–Heaviside equations, are a set of coupled partial differential equations that, together with the Lorentz force law, form the foundation of classical electromagnetism, classical optics, and electric circuits. The equations provide a mathematical model for electric, optical, and radio technologies, such as power generation, electric motors, wireless communication, lenses, radar, etc. They describe how electric and magnetic fields are generated by charges, currents, and changes of the fields. The equations are named after the physicist and mathematician James Clerk Maxwell, who, in 1861 and 1862, published an early form of the equations that included the Lorentz force law. Maxwell first used the equations to propose that light is an electromagnetic phenomenon. The modern form of the equations in their most common formulation is credited to Oliver Heaviside.

A magnetic field is a vector field that describes the magnetic influence on moving electric charges, electric currents, and magnetic materials. A moving charge in a magnetic field experiences a force perpendicular to its own velocity and to the magnetic field. A permanent magnet's magnetic field pulls on ferromagnetic materials such as iron, and attracts or repels other magnets. In addition, a nonuniform magnetic field exerts minuscule forces on "nonmagnetic" materials by three other magnetic effects: paramagnetism, diamagnetism, and antiferromagnetism, although these forces are usually so small they can only be detected by laboratory equipment. Magnetic fields surround magnetized materials, electric currents, and electric fields varying in time. Since both strength and direction of a magnetic field may vary with location, it is described mathematically by a function assigning a vector to each point of space, called a vector field.

Magnetohydrodynamics is a model of electrically conducting fluids that treats all interpenetrating particle species together as a single continuous medium. It is primarily concerned with the low-frequency, large-scale, magnetic behavior in plasmas and liquid metals and has applications in numerous fields including geophysics, astrophysics, and engineering.

The electric potential is defined as the amount of work energy needed per unit of electric charge to move this charge from a reference point to the specific point in an electric field. More precisely, it is the energy per unit charge for a test charge that is so small that the disturbance of the field under consideration is negligible. The motion across the field is supposed to proceed with negligible acceleration, so as to avoid the test charge acquiring kinetic energy or producing radiation. By definition, the electric potential at the reference point is zero units. Typically, the reference point is earth or a point at infinity, although any point can be used.

In physics, specifically electromagnetism, the magnetic flux through a surface is the surface integral of the normal component of the magnetic field B over that surface. It is usually denoted $Φ$ or $Φ B$ . The SI unit of magnetic flux is the weber, and the CGS unit is the maxwell. Magnetic flux is usually measured with a fluxmeter, which contains measuring coils, and it calculates the magnetic flux from the change of voltage on the coils.

In physics, Gauss's law, also known as Gauss's flux theorem, is a law relating the distribution of electric charge to the resulting electric field. In its integral form, it states that the flux of the electric field out of an arbitrary closed surface is proportional to the electric charge enclosed by the surface, irrespective of how that charge is distributed. Even though the law alone is insufficient to determine the electric field across a surface enclosing any charge distribution, this may be possible in cases where symmetry mandates uniformity of the field. Where no such symmetry exists, Gauss's law can be used in its differential form, which states that the divergence of the electric field is proportional to the local density of charge.

In physics, specifically electromagnetism, the Biot–Savart law is an equation describing the magnetic field generated by a constant electric current. It relates the magnetic field to the magnitude, direction, length, and proximity of the electric current.

In classical electromagnetism, Ampère's circuital law relates the circulation of a magnetic field around a closed loop to the electric current passing through the loop.

In vector calculus a solenoidal vector field is a vector field v with divergence zero at all points in the field:

In physics, chemistry and biology, a potential gradient is the local rate of change of the potential with respect to displacement, i.e. spatial derivative, or gradient. This quantity frequently occurs in equations of physical processes because it leads to some form of flux.

In electromagnetism, displacement current density is the quantity $\partial D /\partial t$ appearing in Maxwell's equations that is defined in terms of the rate of change of $D$ , the electric displacement field. Displacement current density has the same units as electric current density, and it is a source of the magnetic field just as actual current is. However it is not an electric current of moving charges, but a time-varying electric field. In physical materials, there is also a contribution from the slight motion of charges bound in atoms, called dielectric polarization.

"A Dynamical Theory of the Electromagnetic Field" is a paper by James Clerk Maxwell on electromagnetism, published in 1865. In the paper, Maxwell derives an electromagnetic wave equation with a velocity for light in close agreement with measurements made by experiment, and deduces that light is an electromagnetic wave.

In classical electromagnetism, magnetic vector potential is the vector quantity defined so that its curl is equal to the magnetic field: $. Together with the electric potential φ, the magnetic vector potential can be used to specify the electric field E as well. Therefore, many equations of electromagnetism can be written either in terms of the fields E and B, or equivalently in terms of the potentials φ and A . In more advanced theories such as quantum mechanics, most equations use potentials rather than fields.$

A classical field theory is a physical theory that predicts how one or more physical fields interact with matter through field equations, without considering effects of quantization; theories that incorporate quantum mechanics are called quantum field theories. In most contexts, 'classical field theory' is specifically intended to describe electromagnetism and gravitation, two of the fundamental forces of nature.

Magnetostatics is the study of magnetic fields in systems where the currents are steady. It is the magnetic analogue of electrostatics, where the charges are stationary. The magnetization need not be static; the equations of magnetostatics can be used to predict fast magnetic switching events that occur on time scales of nanoseconds or less. Magnetostatics is even a good approximation when the currents are not static – as long as the currents do not alternate rapidly. Magnetostatics is widely used in applications of micromagnetics such as models of magnetic storage devices as in computer memory.

A flux tube is a generally tube-like (cylindrical) region of space containing a magnetic field, B, such that the cylindrical sides of the tube are everywhere parallel to the magnetic field lines. It is a graphical visual aid for visualizing a magnetic field. Since no magnetic flux passes through the sides of the tube, the flux through any cross section of the tube is equal, and the flux entering the tube at one end is equal to the flux leaving the tube at the other. Both the cross-sectional area of the tube and the magnetic field strength may vary along the length of the tube, but the magnetic flux inside is always constant.

Computational magnetohydrodynamics (CMHD) is a rapidly developing branch of magnetohydrodynamics that uses numerical methods and algorithms to solve and analyze problems that involve electrically conducting fluids. Most of the methods used in CMHD are borrowed from the well established techniques employed in Computational fluid dynamics. The complexity mainly arises due to the presence of a magnetic field and its coupling with the fluid. One of the important issues is to numerically maintain the $(conservation of magnetic flux) condition, from Maxwell's equations, to avoid the presence of unrealistic effects, namely magnetic monopoles, in the solutions.$

In physics, Gauss's law for gravity, also known as Gauss's flux theorem for gravity, is a law of physics that is equivalent to Newton's law of universal gravitation. It is named after Carl Friedrich Gauss. It states that the flux of the gravitational field over any closed surface is proportional to the mass enclosed. Gauss's law for gravity is often more convenient to work from than Newton's law.

Magnetohydrodynamic turbulence concerns the chaotic regimes of magnetofluid flow at high Reynolds number. Magnetohydrodynamics (MHD) deals with what is a quasi-neutral fluid with very high conductivity. The fluid approximation implies that the focus is on macro length-and-time scales which are much larger than the collision length and collision time respectively.

In ideal magnetohydrodynamics, Alfvén's theorem, or the frozen-in flux theorem, states that electrically conducting fluids and embedded magnetic fields are constrained to move together in the limit of large magnetic Reynolds numbers. It is named after Hannes Alfvén, who put the idea forward in 1943.

References

1 2 Chow, Tai L. (2006). Electromagnetic Theory: A modern perspective. Jones and Bartlett. p. 134. ISBN 0-7637-3827-1.
1 2 Jackson, John David (1999). Classical Electrodynamics (3rd ed.). Wiley. p. 237. ISBN 0-471-30932-X.
↑ Joannopoulos, John D.; Johnson, Steve G.; Winn, Joshua N.; Meade, Robert D. (2008). Photonic Crystals: Molding the Flow of Light (2nd ed.). Princeton University Press. p. 9. ISBN 978-0-691-12456-8.
↑ Schilders, W. H. A.; et al. (2005). Handbook of Numerical Analysis. Elsevier Science. p. 13. ISBN 978-0-444-51375-5.^{[ permanent dead link ]}
↑ Jackson, John David (1999). Classical Electrodynamics (3rd ed.). Wiley. p. 180. ISBN 0-471-30932-X.
↑ Jackson, John David (1999). Classical Electrodynamics (3rd ed.). Wiley. p. 273, eq. 6.150.
↑ See for example equation 4 in Nowakowski, M.; Kelkar, N. G. (2005). "Faraday's law in the presence of magnetic monopoles". Europhysics Letters . 71 (3): 346. arXiv: physics/0508099 . Bibcode:2005EL.....71..346N. doi:10.1209/epl/i2004-10545-2. S2CID 17729781.
↑ Moulin, F. (2001). "Magnetic monopoles and Lorentz force". Il Nuovo Cimento B . 116 (8): 869–877. arXiv: math-ph/0203043 . Bibcode:2001NCimB.116..869M.
↑ Magnetic Monopoles, report from Particle data group, updated August 2015 by D. Milstead and E.J. Weinberg. "To date there have been no confirmed observations of exotic particles possessing magnetic charge."
↑ Castelnovo, C.; Moessner, R.; Sondhi, S. L. (January 3, 2008). "Magnetic monopoles in spin ice". Nature. 451 (7174): 42–45. arXiv:0710.5515. Bibcode:2008Natur.451...42C. doi:10.1038/nature06433. PMID 18172493. S2CID 2399316.
↑ Brackbill, J.U; Barnes, D.C (May 1980). "The Effect of Nonzero ∇ · B on the numerical solution of the magnetohydrodynamic equations". Journal of Computational Physics. 35 (3): 426–430. Bibcode:1980JCoPh..35..426B. doi:10.1016/0021-9991(80)90079-0.
↑ Tóth, Gábor (1 July 2000). "The ∇·B=0 Constraint in Shock-Capturing Magnetohydrodynamics Codes". Journal of Computational Physics. 161 (2): 605–652. Bibcode:2000JCoPh.161..605T. doi:10.1006/jcph.2000.6519. ISSN 0021-9991. S2CID 122112157.
↑ Hernquist, Lars; Vogelsberger, Mark; Mocz, Philip (21 July 2014). "A constrained transport scheme for MHD on unstructured static and moving meshes". Monthly Notices of the Royal Astronomical Society. 442 (1): 43–55. arXiv: 1402.5963 . Bibcode:2014MNRAS.442...43M. doi: 10.1093/mnras/stu865 . ISSN 0035-8711.
↑ Jardin, Stephen (2010). Computational Methods in Plasma Physics (1st ed.). Boca Raton: CRC Press. ISBN 9780429075537.
↑ Hu, Kaibo; Ma, Yicong; Xu, Jinchao (1 February 2017). "Stable finite element methods preserving ∇·B=0 exactly for MHD models". Numerische Mathematik. 135 (2): 371–396. doi:10.1007/s00211-016-0803-4. ISSN 0945-3245. S2CID 30546761.
↑ Ma, Yicong; Hu, Kaibo; Hu, Xiaozhe; Xu, Jinchao (July 2016). "Robust preconditioners for incompressible MHD models". Journal of Computational Physics. 316: 721–746. arXiv: 1503.02553 . Bibcode:2016JCoPh.316..721M. doi:10.1016/j.jcp.2016.04.019. S2CID 7777728.

External links

Media related to Gauss's law for magnetism at Wikimedia Commons

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[Chow-1] 1 2 Chow, Tai L. (2006). Electromagnetic Theory: A modern perspective. Jones and Bartlett. p. 134. ISBN 0-7637-3827-1.

[Jackson-2] 1 2 Jackson, John David (1999). Classical Electrodynamics (3rd ed.). Wiley. p. 237. ISBN 0-471-30932-X.

[Joannopoulos-3] Joannopoulos, John D.; Johnson, Steve G.; Winn, Joshua N.; Meade, Robert D. (2008). Photonic Crystals: Molding the Flow of Light (2nd ed.). Princeton University Press. p. 9. ISBN 978-0-691-12456-8.

[4] Schilders, W. H. A.; et al. (2005). Handbook of Numerical Analysis. Elsevier Science. p. 13. ISBN 978-0-444-51375-5.^{[ permanent dead link ]}

[5] Jackson, John David (1999). Classical Electrodynamics (3rd ed.). Wiley. p. 180. ISBN 0-471-30932-X.

[6] Jackson, John David (1999). Classical Electrodynamics (3rd ed.). Wiley. p. 273, eq. 6.150.

[7] See for example equation 4 in Nowakowski, M.; Kelkar, N. G. (2005). "Faraday's law in the presence of magnetic monopoles". Europhysics Letters . 71 (3): 346. arXiv: physics/0508099 . Bibcode:2005EL.....71..346N. doi:10.1209/epl/i2004-10545-2. S2CID 17729781.

[8] Moulin, F. (2001). "Magnetic monopoles and Lorentz force". Il Nuovo Cimento B . 116 (8): 869–877. arXiv: math-ph/0203043 . Bibcode:2001NCimB.116..869M.

[9] Magnetic Monopoles, report from Particle data group, updated August 2015 by D. Milstead and E.J. Weinberg. "To date there have been no confirmed observations of exotic particles possessing magnetic charge."

[10] Castelnovo, C.; Moessner, R.; Sondhi, S. L. (January 3, 2008). "Magnetic monopoles in spin ice". Nature. 451 (7174): 42–45. arXiv:0710.5515. Bibcode:2008Natur.451...42C. doi:10.1038/nature06433. PMID 18172493. S2CID 2399316.

[11] Brackbill, J.U; Barnes, D.C (May 1980). "The Effect of Nonzero ∇ · B on the numerical solution of the magnetohydrodynamic equations". Journal of Computational Physics. 35 (3): 426–430. Bibcode:1980JCoPh..35..426B. doi:10.1016/0021-9991(80)90079-0.

[12] Tóth, Gábor (1 July 2000). "The ∇·B=0 Constraint in Shock-Capturing Magnetohydrodynamics Codes". Journal of Computational Physics. 161 (2): 605–652. Bibcode:2000JCoPh.161..605T. doi:10.1006/jcph.2000.6519. ISSN 0021-9991. S2CID 122112157.

[13] Hernquist, Lars; Vogelsberger, Mark; Mocz, Philip (21 July 2014). "A constrained transport scheme for MHD on unstructured static and moving meshes". Monthly Notices of the Royal Astronomical Society. 442 (1): 43–55. arXiv: 1402.5963 . Bibcode:2014MNRAS.442...43M. doi: 10.1093/mnras/stu865 . ISSN 0035-8711.

[14] Jardin, Stephen (2010). Computational Methods in Plasma Physics (1st ed.). Boca Raton: CRC Press. ISBN 9780429075537.

[15] Hu, Kaibo; Ma, Yicong; Xu, Jinchao (1 February 2017). "Stable finite element methods preserving ∇·B=0 exactly for MHD models". Numerische Mathematik. 135 (2): 371–396. doi:10.1007/s00211-016-0803-4. ISSN 0945-3245. S2CID 30546761.

[16] Ma, Yicong; Hu, Kaibo; Hu, Xiaozhe; Xu, Jinchao (July 2016). "Robust preconditioners for incompressible MHD models". Journal of Computational Physics. 316: 721–746. arXiv: 1503.02553 . Bibcode:2016JCoPh.316..721M. doi:10.1016/j.jcp.2016.04.019. S2CID 7777728.

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]

[12]

[13]

[14]

[15]

[16]