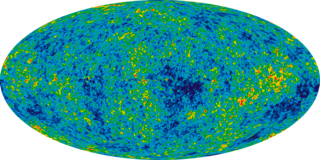
In physical cosmology, cosmic inflation, cosmological inflation, or just inflation, is a theory of exponential expansion of space in the very early universe. Following the inflationary period, the universe continued to expand, but at a slower rate. The re-acceleration of this slowing expansion due to dark energy began after the universe was already over 7.7 billion years old.

In physics, Kaluza–Klein theory is a classical unified field theory of gravitation and electromagnetism built around the idea of a fifth dimension beyond the common 4D of space and time and considered an important precursor to string theory. In their setup, the vacuum has the usual 3 dimensions of space and one dimension of time but with another microscopic extra spatial dimension in the shape of a tiny circle. Gunnar Nordström had an earlier, similar idea. But in that case, a fifth component was added to the electromagnetic vector potential, representing the Newtonian gravitational potential, and writing the Maxwell equations in five dimensions.
In mathematics and physics, a scalar field is a function associating a single number to each point in a region of space – possibly physical space. The scalar may either be a pure mathematical number (dimensionless) or a scalar physical quantity.

Brane cosmology refers to several theories in particle physics and cosmology related to string theory, superstring theory and M-theory.
In physics, the term swampland refers to effective low-energy physical theories which are not compatible with quantum gravity. This is in contrast with the so-called "string theory landscape" that are known to be compatible with string theory, which is hypothesized to be a consistent quantum theory of gravity. In other words, the Swampland is the set of consistent-looking theories with no consistent ultraviolet completion with the addition of gravity.
In theoretical physics, type II string theory is a unified term that includes both type IIA strings and type IIB strings theories. Type II string theory accounts for two of the five consistent superstring theories in ten dimensions. Both theories have extended supersymmetry which is maximal amount of supersymmetry — namely 32 supercharges — in ten dimensions. Both theories are based on oriented closed strings. On the worldsheet, they differ only in the choice of GSO projection. They were first discovered by Michael Green and John Henry Schwarz in 1982, with the terminology of type I and type II coined to classify the three string theories known at the time.
In physics, the Brans–Dicke theory of gravitation is a competitor to Einstein's general theory of relativity. It is an example of a scalar–tensor theory, a gravitational theory in which the gravitational interaction is mediated by a scalar field as well as the tensor field of general relativity. The gravitational constant is not presumed to be constant but instead is replaced by a scalar field which can vary from place to place and with time.
In theoretical physics, the hypothetical particle called the graviscalar or radion emerges as an excitation of general relativity's metric tensor, i.e. gravitational field, but is indistinguishable from a scalar in four dimensions, as shown in Kaluza–Klein theory. The scalar field comes from a component of the metric tensor where the figure 5 labels an additional fifth dimension. The only variations in the scalar field represent variations in the size of the extra dimension. Also, in models with multiple extra dimensions, there exist several such particles. Moreover, in theories with extended supersymmetry, a graviscalar is usually a superpartner of the graviton that behaves as a particle with spin 0. This concept closely relates to the gauged Higgs models.
Higher-dimensional Einstein gravity is any of various physical theories that attempt to generalise to higher dimensions various results of the well established theory of standard (four-dimensional) Albert Einstein's gravitational theory, that is, general relativity. This attempt at generalisation has been strongly influenced in recent decades by string theory.
String cosmology is a relatively new field that tries to apply equations of string theory to solve the questions of early cosmology. A related area of study is brane cosmology.
In theoretical physics, massive gravity is a theory of gravity that modifies general relativity by endowing the graviton with a nonzero mass. In the classical theory, this means that gravitational waves obey a massive wave equation and hence travel at speeds below the speed of light.
In theoretical physics, a scalar–tensor theory is a field theory that includes both a scalar field and a tensor field to represent a certain interaction. For example, the Brans–Dicke theory of gravitation uses both a scalar field and a tensor field to mediate the gravitational interaction.
Dimensional reduction is the limit of a compactified theory where the size of the compact dimension goes to zero. In physics, a theory in D spacetime dimensions can be redefined in a lower number of dimensions d, by taking all the fields to be independent of the location in the extra D − d dimensions.

The causal sets program is an approach to quantum gravity. Its founding principles are that spacetime is fundamentally discrete and that spacetime events are related by a partial order. This partial order has the physical meaning of the causality relations between spacetime events.
The BTZ black hole, named after Máximo Bañados, Claudio Teitelboim, and Jorge Zanelli, is a black hole solution for (2+1)-dimensional topological gravity with a negative cosmological constant.
The Callan–Giddings–Harvey–Strominger model or CGHS model in short is a toy model of general relativity in 1 spatial and 1 time dimension.
Jackiw–Teitelboim gravity, also known as the R = T model, or simply JT gravity, is a theory of gravity with dilaton coupling in one spatial and one time dimension. It should not be confused with the CGHS model or Liouville gravity. The action is given by
In physics, Liouville field theory is a two-dimensional conformal field theory whose classical equation of motion is a generalization of Liouville's equation.

Asymptotic safety is a concept in quantum field theory which aims at finding a consistent and predictive quantum theory of the gravitational field. Its key ingredient is a nontrivial fixed point of the theory's renormalization group flow which controls the behavior of the coupling constants in the ultraviolet (UV) regime and renders physical quantities safe from divergences. Although originally proposed by Steven Weinberg to find a theory of quantum gravity, the idea of a nontrivial fixed point providing a possible UV completion can be applied also to other field theories, in particular to perturbatively nonrenormalizable ones. In this respect, it is similar to quantum triviality.
The pressuron is a hypothetical scalar particle which couples to both gravity and matter theorised in 2013. Although originally postulated without self-interaction potential, the pressuron is also a dark energy candidate when it has such a potential. The pressuron takes its name from the fact that it decouples from matter in pressure-less regimes, allowing the scalar–tensor theory of gravity involving it to pass solar system tests, as well as tests on the equivalence principle, even though it is fundamentally coupled to matter. Such a decoupling mechanism could explain why gravitation seems to be well described by general relativity at present epoch, while it could actually be more complex than that. Because of the way it couples to matter, the pressuron is a special case of the hypothetical string dilaton. Therefore, it is one of the possible solutions to the present non-observation of various signals coming from massless or light scalar fields that are generically predicted in string theory.






