
The stress–energy tensor, sometimes called the stress–energy–momentum tensor or the energy–momentum tensor, is a tensor physical quantity that describes the density and flux of energy and momentum in spacetime, generalizing the stress tensor of Newtonian physics. It is an attribute of matter, radiation, and non-gravitational force fields. This density and flux of energy and momentum are the sources of the gravitational field in the Einstein field equations of general relativity, just as mass density is the source of such a field in Newtonian gravity.

In mathematics and theoretical physics, the term quantum group denotes one of a few different kinds of noncommutative algebras with additional structure. These include Drinfeld–Jimbo type quantum groups, compact matrix quantum groups, and bicrossproduct quantum groups. Despite their name, they do not themselves have a natural group structure, though they are in some sense 'close' to a group.
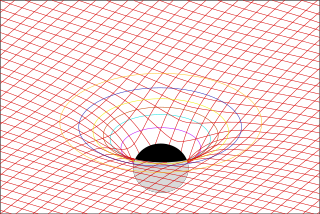
In the general theory of relativity, the Einstein field equations relate the geometry of spacetime to the distribution of matter within it.
In differential geometry, the Einstein tensor is used to express the curvature of a pseudo-Riemannian manifold. In general relativity, it occurs in the Einstein field equations for gravitation that describe spacetime curvature in a manner that is consistent with conservation of energy and momentum.
In differential geometry, a tensor density or relative tensor is a generalization of the tensor field concept. A tensor density transforms as a tensor field when passing from one coordinate system to another, except that it is additionally multiplied or weighted by a power W of the Jacobian determinant of the coordinate transition function or its absolute value. A tensor density with a single index is called a vector density. A distinction is made among (authentic) tensor densities, pseudotensor densities, even tensor densities and odd tensor densities. Sometimes tensor densities with a negative weight W are called tensor capacity. A tensor density can also be regarded as a section of the tensor product of a tensor bundle with a density bundle.

In electromagnetism, the electromagnetic tensor or electromagnetic field tensor is a mathematical object that describes the electromagnetic field in spacetime. The field tensor was first used after the four-dimensional tensor formulation of special relativity was introduced by Hermann Minkowski. The tensor allows related physical laws to be written concisely, and allows for the quantization of the electromagnetic field by the Lagrangian formulation described below.
In general relativity, a geodesic generalizes the notion of a "straight line" to curved spacetime. Importantly, the world line of a particle free from all external, non-gravitational forces is a particular type of geodesic. In other words, a freely moving or falling particle always moves along a geodesic.
In theoretical physics, massive gravity is a theory of gravity that modifies general relativity by endowing the graviton with a nonzero mass. In the classical theory, this means that gravitational waves obey a massive wave equation and hence travel at speeds below the speed of light.

The covariant formulation of classical electromagnetism refers to ways of writing the laws of classical electromagnetism in a form that is manifestly invariant under Lorentz transformations, in the formalism of special relativity using rectilinear inertial coordinate systems. These expressions both make it simple to prove that the laws of classical electromagnetism take the same form in any inertial coordinate system, and also provide a way to translate the fields and forces from one frame to another. However, this is not as general as Maxwell's equations in curved spacetime or non-rectilinear coordinate systems.
In physics, Maxwell's equations in curved spacetime govern the dynamics of the electromagnetic field in curved spacetime or where one uses an arbitrary coordinate system. These equations can be viewed as a generalization of the vacuum Maxwell's equations which are normally formulated in the local coordinates of flat spacetime. But because general relativity dictates that the presence of electromagnetic fields induce curvature in spacetime, Maxwell's equations in flat spacetime should be viewed as a convenient approximation.
In the theory of general relativity, a stress–energy–momentum pseudotensor, such as the Landau–Lifshitz pseudotensor, is an extension of the non-gravitational stress–energy tensor that incorporates the energy–momentum of gravity. It allows the energy–momentum of a system of gravitating matter to be defined. In particular it allows the total of matter plus the gravitating energy–momentum to form a conserved current within the framework of general relativity, so that the total energy–momentum crossing the hypersurface of any compact space–time hypervolume vanishes.
The Newman–Penrose (NP) formalism is a set of notation developed by Ezra T. Newman and Roger Penrose for general relativity (GR). Their notation is an effort to treat general relativity in terms of spinor notation, which introduces complex forms of the usual variables used in GR. The NP formalism is itself a special case of the tetrad formalism, where the tensors of the theory are projected onto a complete vector basis at each point in spacetime. Usually this vector basis is chosen to reflect some symmetry of the spacetime, leading to simplified expressions for physical observables. In the case of the NP formalism, the vector basis chosen is a null tetrad: a set of four null vectors—two real, and a complex-conjugate pair. The two real members often asymptotically point radially inward and radially outward, and the formalism is well adapted to treatment of the propagation of radiation in curved spacetime. The Weyl scalars, derived from the Weyl tensor, are often used. In particular, it can be shown that one of these scalars— in the appropriate frame—encodes the outgoing gravitational radiation of an asymptotically flat system.
In mathematics, Ricci calculus constitutes the rules of index notation and manipulation for tensors and tensor fields on a differentiable manifold, with or without a metric tensor or connection. It is also the modern name for what used to be called the absolute differential calculus, tensor calculus or tensor analysis developed by Gregorio Ricci-Curbastro in 1887–1896, and subsequently popularized in a paper written with his pupil Tullio Levi-Civita in 1900. Jan Arnoldus Schouten developed the modern notation and formalism for this mathematical framework, and made contributions to the theory, during its applications to general relativity and differential geometry in the early twentieth century. The basis of modern tensor analysis was developed by Bernhard Riemann in a paper from 1861.
In the Newman–Penrose (NP) formalism of general relativity, independent components of the Ricci tensors of a four-dimensional spacetime are encoded into seven Ricci scalars which consist of three real scalars , three complex scalars and the NP curvature scalar . Physically, Ricci-NP scalars are related with the energy–momentum distribution of the spacetime due to Einstein's field equation.
Affine gauge theory is classical gauge theory where gauge fields are affine connections on the tangent bundle over a smooth manifold . For instance, these are gauge theory of dislocations in continuous media when , the generalization of metric-affine gravitation theory when is a world manifold and, in particular, gauge theory of the fifth force.
In theoretical physics, the Curtright field (named after Thomas Curtright) is a tensor quantum field of mixed symmetry, whose gauge-invariant dynamics are dual to those of the general relativistic graviton in higher (D>4) spacetime dimensions. Or at least this holds for the linearized theory. For the full nonlinear theory, less is known. Several difficulties arise when interactions of mixed symmetry fields are considered, but at least in situations involving an infinite number of such fields (notably string theory) these difficulties are not insurmountable.
Bimetric gravity or bigravity refers to two different classes of theories. The first class of theories relies on modified mathematical theories of gravity in which two metric tensors are used instead of one. The second metric may be introduced at high energies, with the implication that the speed of light could be energy-dependent, enabling models with a variable speed of light.

In relativistic quantum mechanics and quantum field theory, the Joos–Weinberg equation is a relativistic wave equation applicable to free particles of arbitrary spin j, an integer for bosons or half-integer for fermions. The solutions to the equations are wavefunctions, mathematically in the form of multi-component spinor fields. The spin quantum number is usually denoted by s in quantum mechanics, however in this context j is more typical in the literature.
In supersymmetry, type IIA supergravity is the unique supergravity in ten dimensions with two supercharges of opposite chirality. It was first constructed in 1984 by a dimensional reduction of eleven-dimensional supergravity on a circle. The other supergravities in ten dimensions are type IIB supergravity, which has two supercharges of the same chirality, and type I supergravity, which has a single supercharge. In 1986 a deformation of the theory was discovered which gives mass to one of the fields and is known as massive type IIA supergravity. Type IIA supergravity plays a very important role in string theory as it is the low-energy limit of type IIA string theory.
In supersymmetry, type IIB supergravity is the unique supergravity in ten dimensions with two supercharges of the same chirality. It was first constructed in 1983 by John Schwarz and independently by Paul Howe and Peter West at the level of its equations of motion. While it does not admit a fully covariant action due to the presence of a self-dual field, it can be described by an action if the self-duality condition is imposed by hand on the resulting equations of motion. The other types of supergravity in ten dimensions are type IIA supergravity, which has two supercharges of opposing chirality, and type I supergravity, which has a single supercharge. The theory plays an important role in modern physics since it is the low-energy limit of type IIB string theory.






















































