Metabolic theory of ecology

Last updated

The metabolic theory of ecology (MTE) [1] is the ecological component of the more general Metabolic Scaling Theory [2] and Kleiber's law. It posits that the metabolic rate of organisms is the fundamental biological rate that governs most observed patterns in ecology. MTE is part of a larger set of theory known as metabolic scaling theory that attempts to provide a unified theory for the importance of metabolism in driving pattern and process in biology from the level of cells all the way to the biosphere. [2] [3] [4]

Contents

MTE is based on an interpretation of the relationships between body size, body temperature, and metabolic rate across all organisms. Small-bodied organisms tend to have higher mass-specific metabolic rates than larger-bodied organisms. Furthermore, organisms that operate at warm temperatures through endothermy or by living in warm environments tend towards higher metabolic rates than organisms that operate at colder temperatures. This pattern is consistent from the unicellular level up to the level of the largest animals and plants on the planet.

In MTE, this relationship is considered to be the primary constraint that influences biological processes (via their rates and times) at all levels of organization (from individual up to ecosystem level). MTE is a macroecological theory that aims to be universal in scope and application. [1] [5]

Fundamental concepts in MTE

Metabolism

Metabolic pathways consist of complex networks, which are responsible for the processing of both energy and material. The metabolic rate of a heterotroph is defined as the rate of respiration in which energy is obtained by oxidation of carbon compound. The rate of photosynthesis on the other hand, indicates the metabolic rate of an autotroph. [6] According to MTE, both body size and temperature affect the metabolic rate of an organism. Metabolic rate scale as 3/4 power of body size, and its relationship with temperature is described by Van’t Hoff-Arrhenius equation over the range of 0 to 40 °C. [7]

Stoichiometry

From the ecological perspective, stoichiometry is concerned with the proportion of elements in both living organisms and their environment. [8] In order to survive and maintain metabolism, an organism must be able to obtain crucial elements and excrete waste products. As a result, the elemental composition of an organism would be different from the exterior environment. [9] Through metabolism, body size can affect stoichiometry. For example, small organism tend to store most of their phosphorus in rRNA due to their high metabolic rate, [10] [11] [12] whereas large organisms mostly invest this element inside the skeletal structure. Thus, concentration of elements to some extent can limit the rate of biological processes. Inside an ecosystem, the rate of flux and turn over of elements by inhabitants, combined with the influence of abiotic factors, determine the concentration of elements. [1]

Theoretical background

Metabolic rate scales with the mass of an organism of a given species according to Kleiber's law where B is whole organism metabolic rate (in watts or other unit of power), M is organism mass (in kg), and Bo is a mass-independent normalization constant (given in a unit of power divided by a unit of mass. In this case, watts per kilogram):

At increased temperatures, chemical reactions proceed faster. This relationship is described by the Boltzmann factor, where E is activation energy in electronvolts or joules, T is absolute temperature in kelvins, and k is the Boltzmann constant in eV/K or J/K:

While Bo in the previous equation is mass-independent, it is not explicitly independent of temperature. To explain the relationship between body mass and temperature, building on earlier work [13] showing that the effects of both body mass and temperature could be combined multiplicatively in a single equation, the two equations above can be combined to produce the primary equation of the MTE, where bo is a normalization constant that is independent of body size or temperature:

According to this relationship, metabolic rate is a function of an organism's body mass and body temperature. By this equation, large organisms have higher metabolic rates (in watts) than small organisms, and organisms at high body temperatures have higher metabolic rates than those that exist at low body temperatures. However, specific metabolic rate (SMR, in watts/kg) is given by

Hence SMR for large organisms are lower than small organisms.

Past debate over mechanisms and the allometric exponent

Researchers have debated two main aspects of this theory, the pattern and the mechanism. Past debated have focused on the question whether metabolic rate scales to the power of 3⁄4 or 2⁄3w, or whether either of these can even be considered a universal exponent. [14] [15] [16] In addition to debates concerning the exponent, some researchers also disagree about the underlying mechanisms generating the scaling exponent. Various authors have proposed at least eight different types of mechanisms that predict an allometric scaling exponent of either 2⁄3 or 3⁄4. The majority view is that while the 3⁄4 exponent is indeed the mean observed exponent within and across taxa, there is intra- and interspecific variability in the exponent that can include shallower exponents such as2⁄3. [17] Past debates on the exact value of the exponent are settled in part because the observed variability in the metabolic scaling exponent is consistent with a 'relaxed' version of metabolic scaling theory where additional selective pressures lead to a constrained set of variation around the predicted optimal 3⁄4 exponent. [18]

Much of past debate have focused on two particular types of mechanisms. [16] One of these assumes energy or resource transport across the external surface area of three-dimensional organisms is the key factor driving the relationship between metabolic rate and body size. The surface area in question may be skin, lungs, intestines, or, in the case of unicellular organisms, cell membranes. In general, the surface area (SA) of a three dimensional object scales with its volume (V) as SA = cV2⁄3, where c is a proportionality constant. The Dynamic Energy Budget model predicts exponents that vary between 2⁄3 – 1, depending on the organism's developmental stage, basic body plan and resource density. [19] [20] DEB is an alternative to metabolic scaling theory, developed before the MTE. [21] DEB also provides a basis for population, community and ecosystem level processes to be studied based on energetics of the constituent organisms. In this theory, the biomass of the organism is separated into structure (what is built during growth) and reserve (a pool of polymers generated by assimilation). DEB is based on the first principles dictated by the kinetics and thermodynamics of energy and material fluxes, has a similar number of parameters per process as MTE, [22] and the parameters have been estimated for over 3000 animal species "Add my Pet" . Retrieved 23 August 2022. While some of these alternative models make several testable predictions, others are less comprehensive [15] and of these proposed models only DEB can make as many predictions with a minimal set of assumptions as metabolic scaling theory. [21]

In contrast, the arguments for a 3⁄4 scaling factor are based on resource transport network models, [16] where the limiting resources are distributed via some optimized network to all resource consuming cells or organelles. [2] [23] These models are based on the assumption that metabolism is proportional to the rate at which an organism's distribution networks (such as circulatory systems in animals or xylem and phloem in plants) deliver nutrients and energy to body tissues. [2] [24] [25] Larger organisms are necessarily less efficient because more resource is in transport at any one time than in smaller organisms: size of the organism and length of the network imposes an inefficiency due to size. It therefore takes somewhat longer for large organisms to distribute nutrients throughout the body and thus they have a slower mass-specific metabolic rate. An organism that is twice as large cannot metabolize twice the energy—it simply has to run more slowly because more energy and resources are wasted being in transport, rather than being processed. Nonetheless, natural selection appears to have minimized this inefficiency by favoring resource transport networks that maximize rate of delivery of resources to the end points such as cells and organelles. [23] [24] This selection to maximize metabolic rate and energy dissipation results in the allometric exponent that tends to D/(D+1), where D is the primary dimension of the system. A three dimensional system, such as an individual, tends to scale to the 3/4 power, whereas a two dimensional network, such as a river network in a landscape, tends to scale to the 2/3 power. [23] [25] [26]

Despite past debates over the value of the exponent, the implications of metabolic scaling theory and the extensions of the theory to ecology (metabolic theory of ecology) the theory might remain true regardless of its precise numerical value.

Implications of the theory

The metabolic theory of ecology's main implication is that metabolic rate, and the influence of body size and temperature on metabolic rate, provide the fundamental constraints by which ecological processes are governed. If this holds true from the level of the individual up to ecosystem level processes, then life history attributes, population dynamics, and ecosystem processes could be explained by the relationship between metabolic rate, body size, and body temperature. While different underlying mechanisms [2] [20] make somewhat different predictions, the following provides an example of some of the implications of the metabolism of individuals.

Organism level

Small animals tend to grow fast, breed early, and die young. [27] According to MTE, these patterns in life history traits are constrained by metabolism. [28] An organism's metabolic rate determines its rate of food consumption, which in turn determines its rate of growth. This increased growth rate produces trade-offs that accelerate senescence. For example, metabolic processes produce free radicals as a by-product of energy production. [29] These in turn cause damage at the cellular level, which promotes senescence and ultimately death. Selection favors organisms which best propagate given these constraints. As a result, smaller, shorter lived organisms tend to reproduce earlier in their life histories.

Population and community level

MTE has profound implications for the interpretation of population growth and community diversity. [27] Classically, species are thought of as being either r selected (where populations tend to grow exponentially, and are ultimately limited by extrinsic factors) or K selected (where population size is limited by density-dependence and carrying capacity). MTE explains this diversity of reproductive strategies as a consequence of the metabolic constraints of organisms. Small organisms and organisms that exist at high body temperatures tend to be r selected, which fits with the prediction that r selection is a consequence of metabolic rate. [1] Conversely, larger and cooler bodied animals tend to be K selected. The relationship between body size and rate of population growth has been demonstrated empirically, [30] and in fact has been shown to scale to M−1/4 across taxonomic groups. [27] The optimal population growth rate for a species is therefore thought to be determined by the allometric constraints outlined by the MTE, rather than strictly as a life history trait that is selected for based on environmental conditions.

Regarding density, MTE predicts carrying capacity of populations to scale as M-3/4, and to exponentially decrease with increasing temperature. The fact that larger organisms reach carrying capacity sooner than smaller one is intuitive, however, temperature can also decrease carrying capacity due to the fact that in warmer environments, higher metabolic rate of organisms demands a higher rate of supply. [31] Empirical evidence in terrestrial plants, also suggests that density scales as -3/4 power of the body size. [32]

Observed patterns of diversity can be similarly explained by MTE. It has long been observed that there are more small species than large species. [33] In addition, there are more species in the tropics than at higher latitudes. [1] Classically, the latitudinal gradient in species diversity has been explained by factors such as higher productivity or reduced seasonality. [34] In contrast, MTE explains this pattern as being driven by the kinetic constraints imposed by temperature on metabolism. [31] The rate of molecular evolution scales with metabolic rate, [35] such that organisms with higher metabolic rates show a higher rate of change at the molecular level. [1] If a higher rate of molecular evolution causes increased speciation rates, then adaptation and ultimately speciation may occur more quickly in warm environments and in small bodied species, ultimately explaining observed patterns of diversity across body size and latitude.

MTE's ability to explain patterns of diversity remains controversial. For example, researchers analyzed patterns of diversity of New World coral snakes to see whether the geographical distribution of species fit within the predictions of MTE (i.e. more species in warmer areas). [36] They found that the observed pattern of diversity could not be explained by temperature alone, and that other spatial factors such as primary productivity, topographic heterogeneity, and habitat factors better predicted the observed pattern. Extensions of metabolic theory to diversity that include eco-evolutionary theory show that an elaborated metabolic theory can account for differences in diversity gradients by including feedbacks between ecological interactions (size-dependent competition and predation) and evolutionary rates (speciation and extinction) [37]

Ecosystem processes

At the ecosystem level, MTE explains the relationship between temperature and production of total biomass. [38] The average production to biomass ratio of organisms is higher in small organisms than large ones. [39] This relationship is further regulated by temperature, and the rate of production increases with temperature. [40] As production consistently scales with body mass, MTE provides a framework to assess the relative importance of organismal size, temperature, functional traits, soil and climate on variation in rates of production within and across ecosystems. [38] Metabolic theory shows that variation in ecosystem production is characterized by a common scaling relationship, suggesting that global change models can incorporate the mechanisms governing this relationship to improve predictions of future ecosystem function.

See also

Related Research Articles

<span class="mw-page-title-main">Ecology</span> Study of organisms and their environment

Ecology is the study of the relationships among living organisms, including humans, and their physical environment. Ecology considers organisms at the individual, population, community, ecosystem, and biosphere level. Ecology overlaps with the closely related sciences of biogeography, evolutionary biology, genetics, ethology, and natural history.

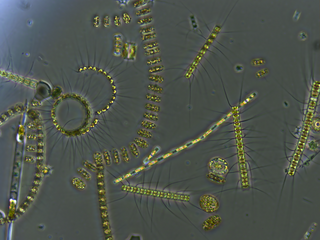
<span class="mw-page-title-main">Zooplankton</span> Heterotrophic protistan or metazoan members of the plankton ecosystem

Zooplankton are the animal component of the planktonic community. Plankton are aquatic organisms that are unable to swim effectively against currents. Consequently, they drift or are carried along by currents in the ocean, or by currents in seas, lakes or rivers.

<span class="mw-page-title-main">Hibernation</span> Physiological state of dormant inactivity in order to pass the winter season

Hibernation is a state of minimal activity and metabolic depression undergone by some animal species. Hibernation is a seasonal heterothermy characterized by low body-temperature, slow breathing and heart-rate, and low metabolic rate. It most commonly occurs during winter months.

Basal metabolic rate (BMR) is the rate of energy expenditure per unit time by endothermic animals at rest. It is reported in energy units per unit time ranging from watt (joule/second) to ml O2/min or joule per hour per kg body mass J/(h·kg). Proper measurement requires a strict set of criteria to be met. These criteria include being in a physically and psychologically undisturbed state and being in a thermally neutral environment while in the post-absorptive state (i.e., not actively digesting food). In bradymetabolic animals, such as fish and reptiles, the equivalent term standard metabolic rate (SMR) applies. It follows the same criteria as BMR, but requires the documentation of the temperature at which the metabolic rate was measured. This makes BMR a variant of standard metabolic rate measurement that excludes the temperature data, a practice that has led to problems in defining "standard" rates of metabolism for many mammals.

The oxygen minimum zone (OMZ), sometimes referred to as the shadow zone, is the zone in which oxygen saturation in seawater in the ocean is at its lowest. This zone occurs at depths of about 200 to 1,500 m (700–4,900 ft), depending on local circumstances. OMZs are found worldwide, typically along the western coast of continents, in areas where an interplay of physical and biological processes concurrently lower the oxygen concentration and restrict the water from mixing with surrounding waters, creating a "pool" of water where oxygen concentrations fall from the normal range of 4–6 mg/L to below 2 mg/L.

James Hemphill Brown is an American biologist and academic.

<span class="mw-page-title-main">Kleiber's law</span>

Kleiber's law, named after Max Kleiber for his biology work in the early 1930s, is the observation that, for the vast majority of animals, an animal's metabolic rate scales to the 3⁄4 power of the animal's mass. More recently, Kleiber's law has also been shown to apply in plants, suggesting that Kleiber's observation is much more general. Symbolically: if B is the animal's metabolic rate, and M is the animal's mass, then Kleiber's law states that B~M3/4. Thus, over the same time span, a cat having a mass 100 times that of a mouse will consume only about 32 times the energy the mouse uses.

<span class="mw-page-title-main">Allometry</span> Study of the relationship of body size to shape, anatomy, physiology, and behavior

Allometry is the study of the relationship of body size to shape, anatomy, physiology and finally behaviour, first outlined by Otto Snell in 1892, by D'Arcy Thompson in 1917 in On Growth and Form and by Julian Huxley in 1932.

The dynamic energy budget (DEB) theory is a formal metabolic theory which provides a single quantitative framework to dynamically describe the aspects of metabolism of all living organisms at the individual level, based on assumptions about energy uptake, storage, and utilization of various substances. The DEB theory adheres to stringent thermodynamic principles, is motivated by universally observed patterns, is non-species specific, and links different levels of biological organization as prescribed by the implications of energetics. Models based on the DEB theory have been successfully applied to over a 1000 species with real-life applications ranging from conservation, aquaculture, general ecology, and ecotoxicology. The theory is contributing to the theoretical underpinning of the emerging field of metabolic ecology.

<span class="mw-page-title-main">Geoffrey West</span>

Geoffrey Brian West is a British theoretical physicist and former president and distinguished professor of the Santa Fe Institute. He is one of the leading scientists working on a scientific model of cities. Among other things, his work states that with the doubling of a city's population, salaries per capita will generally increase by 15%.

<span class="mw-page-title-main">Diel vertical migration</span> A pattern of daily vertical movement characteristic of many aquatic species

Diel vertical migration (DVM), also known as diurnal vertical migration, is a pattern of movement used by some organisms, such as copepods, living in the ocean and in lakes. The word "diel" comes from Latin: diēs, lit. 'day', and means a 24-hour period. The migration occurs when organisms move up to the uppermost layer of the sea at night and return to the bottom of the daylight zone of the oceans or to the dense, bottom layer of lakes during the day. It is important to the functioning of deep-sea food webs and the biologically driven sequestration of carbon.

<span class="mw-page-title-main">Evolutionary physiology</span> Study of changes in physiological characteristics

Evolutionary physiology is the study of the biological evolution of physiological structures and processes; that is, the manner in which the functional characteristics of individuals in a population of organisms have responded to natural selection across multiple generations during the history of the population. It is a sub-discipline of both physiology and evolutionary biology. Practitioners in the field come from a variety of backgrounds, including physiology, evolutionary biology, ecology, and genetics.

<span class="mw-page-title-main">Tree allometry</span> Quantitative relations between some key characteristic dimensions of trees

Tree allometry establishes quantitative relations between some key characteristic dimensions of trees and other properties. To the extent these statistical relations, established on the basis of detailed measurements on a small sample of typical trees, hold for other individuals, they permit extrapolations and estimations of a host of dendrometric quantities on the basis of a single measurements.

An ecological network is a representation of the biotic interactions in an ecosystem, in which species (nodes) are connected by pairwise interactions (links). These interactions can be trophic or symbiotic. Ecological networks are used to describe and compare the structures of real ecosystems, while network models are used to investigate the effects of network structure on properties such as ecosystem stability.

<span class="mw-page-title-main">Deep-sea gigantism</span> Tendency for deep-sea species to be larger than their shallower-water relatives

In zoology, deep-sea gigantism or abyssal gigantism is the tendency for species of invertebrates and other deep-sea dwelling animals to be larger than their shallower-water relatives across a large taxonomic range. Proposed explanations for this type of gigantism include colder temperature, food scarcity, reduced predation pressure and increased dissolved oxygen concentrations in the deep sea. The inaccessibility of abyssal habitats has hindered the study of this topic.

Endothermic organisms known as homeotherms maintain internal temperatures with minimal metabolic regulation within a range of ambient temperatures called the thermal neutral zone (TNZ). Within the TNZ the basal rate of heat production is equal to the rate of heat loss to the environment. Homeothermic organisms adjust to the temperatures within the TNZ through different responses requiring little energy.

<span class="mw-page-title-main">Body size and species richness</span>

The body size-species richness distribution is a pattern observed in the way taxa are distributed over large spatial scales. The number of species that exhibit small body size generally far exceed the number of species that are large-bodied. Macroecology has long sought to understand the mechanisms that underlie the patterns of biodiversity, such as the body size-species richness pattern.

<span class="mw-page-title-main">Rate-of-living theory</span> Theory of biological ageing

The rate of living theory postulates that the faster an organism’s metabolism, the shorter its lifespan. First proposed by Max Rubner in 1908, the theory was based on his observation that smaller animals had faster metabolisms and shorter lifespans compared to larger animals with slower metabolisms. The theory gained further credibility through the work of Raymond Pearl, who conducted experiments on drosophila and cantaloupe seeds, which supported Rubner's initial observation. Pearl's findings were later published in his book, The Rate of Living, in 1928, in which he expounded upon Rubner's theory and demonstrated a causal relationship between the slowing of metabolism and an increase in lifespan.

Brian Joseph Enquist is an American biologist and academic. Enquist is a Professor of Biology at the University of Arizona. He is also external professor at the Santa Fe Institute. He is a biologist, plant biologist and an ecologist. He was elected as a Fellow of the American Association for the Advancement of Science (AAAS) in 2012 and the Ecological Society of America (ESA) in 2018.

<span class="mw-page-title-main">Jarman–Bell principle</span> Ecological concept linking an herbivores diet and size

The Jarman–Bell principle is a concept in ecology that the food quality of a herbivore's intake decreases as the size of the herbivore increases, but the amount of such food increases to counteract the low quality foods. It operates by observing the allometric properties of herbivores. The principle was coined by P.J Jarman (1968.) and R.H.V Bell (1971).

References

  1. 1 2 3 4 5 6 Brown, J. H., Gillooly, J. F., Allen, A. P., Savage, V. M., & G. B. West (2004). "Toward a metabolic theory of ecology". Ecology. 85 (7): 1771–89. doi:10.1890/03-9000. S2CID   691916.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  2. 1 2 3 4 5 West, G. B., Brown, J. H., & Enquist, B. J. (1997). "A general model for the origin of allometric scaling laws in biology". Science. 276 (7): 122–126. doi:10.1126/science.276.5309.122. PMID   9082983. S2CID   3140271.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  3. ↑ Enquist, B.J., Brown, J. H., & West, G. B. (1998). "Allometric scaling of plant energetics and population density". Nature. 395 (6698): 163–165. Bibcode:1998Natur.395..163E. doi:10.1038/25977. PMID   9082983. S2CID   204996904.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  4. ↑ Enquist, B.J., Economo, E.P., Huxman, T.E., Allen, A.P., Ignace, D.D., & Gillooly, J.F. (1997). "Scaling metabolism from organisms to ecosystems". Nature. 423 (6940): 639–642. Bibcode:2003Natur.423..639E. doi: 10.1038/nature01671 . PMID   9082983. S2CID   4426210.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  5. ↑ Enquist, B. J., Economo, E. P., Huxman, T. E., Allen, A. P., Ignace, D. D., & Gillooly, J. F. (2003). "Scaling metabolism from organisms to ecosystems". Nature. 423 (6940): 639–642. Bibcode:2003Natur.423..639E. doi: 10.1038/nature01671 . PMID   12789338. S2CID   4426210.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  6. ↑ Farquhar, G. D.; von Caemmerer, S.; Berry, J. A. (1980). "A biochemical model of photosynthetic CO2 assimilation in leaves of C 3 species". Planta. 149 (1): 78–90. doi:10.1007/BF00386231. ISSN   0032-0935. PMID   24306196. S2CID   20518047.
  7. ↑ Thompson, D'Arcy Wentworth (1992). Bonner, John Tyler (ed.). On Growth and Form. Cambridge Core. doi:10.1017/cbo9781107325852. ISBN   9780521437769 . Retrieved 2019-11-09.
  8. ↑ Redfield, A. C. (1960). "The biological control of chemical factors in the environment". Science Progress. 11: 150–170. ISSN   0036-8504. PMID   24545739.
  9. ↑ "Elements of Physical Biology". Nature. 116 (2917): 461. 1925. Bibcode:1925Natur.116R.461.. doi:10.1038/116461b0. hdl: 2027/mdp.39015078668525 . ISSN   0028-0836. S2CID   4103581.
  10. ↑ Sutcliffe Jr., W. H. (1970-03-01). "Relationship Between Growth Rate and Ribonucleic Acid Concentration in Some Invertebrates". Journal of the Fisheries Research Board of Canada. 27 (3): 606–609. doi:10.1139/f70-065. ISSN   0015-296X.
  11. ↑ Elser, J. J.; Sterner, R. W.; Gorokhova, E.; Fagan, W. F.; Markow, T. A.; Cotner, J. B.; Harrison, J. F.; Hobbie, S. E.; Odell, G. M.; Weider, L. W. (2000-11-23). "Biological stoichiometry from genes to ecosystems". Ecology Letters. 3 (6): 540–550. doi:10.1111/j.1461-0248.2000.00185.x. ISSN   1461-0248.
  12. ↑ Sterner, Robert W.; Elser, James J. (2002-11-17). Ecological Stoichiometry. Princeton University Press. ISBN   9780691074917.
  13. ↑ Robinson, W. R., Peters, R. H., & Zimmermann, J. (1983). "The effects of body size and temperature on metabolic rate of organisms". Canadian Journal of Zoology. 61 (2): 281–288. doi:10.1139/z83-037.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  14. ↑ Tilman, David; HilleRisLambers, Janneke; Harpole, Stan; Dybzinski, Ray; Fargione, Joe; Clark, Chris; Lehman, Clarence (2004). "Does Metabolic Theory Apply to Community Ecology? It's a Matter of Scale". Ecology. 85 (7): 1797–1799. doi: 10.1890/03-0725 . ISSN   0012-9658.
  15. 1 2 Agutter, P.S., Wheatley, D.N. (2004). "Metabolic scaling: consensus or controversy?". Theoretical Biology and Medical Modelling. 1: 1–13. doi: 10.1186/1742-4682-1-13 . PMC   539293 . PMID   15546492.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  16. 1 2 3 Hirst, A. G., Glazier, D. S., & Atkinson, D. (2014). "Body shape shifting during growth permits tests that distinguish between competing geometric theories of metabolic scaling". Ecology Letters. 17 (10): 1274–1281. doi:10.1111/ele.12334. PMID   25060740.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  17. ↑ Vasseur, F., Exposito-Alonso, M., Ayala-Garay, O.J., Wang, G., Enquist, B.J., Vile, D., Violle, C. & Weigel, D. (2018). "Adaptive diversification of growth allometry in the plant Arabidopsis thaliana". PNAS. 115 (13): 3416–3421. doi: 10.1073/pnas.1709141115 . PMC   5879651 . PMID   29540570.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  18. ↑ Price, C.A. Enquist, B.J. & Savage, V.M. (2007). "A general model for allometric covariation in botanical form and function". PNAS. 104 (32): 313204–132091. Bibcode:2007PNAS..10413204P. doi: 10.1073/pnas.0702242104 . PMC   1941814 . PMID   17664421.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  19. ↑ Kooijman, S. A. L. M. (1986). "Energy budgets can explain body size relations". Journal of Theoretical Biology. 121 (3): 269–282. Bibcode:1986JThBi.121..269K. doi:10.1016/s0022-5193(86)80107-2.
  20. 1 2 Kooijman, S. A. L. M. (2010). "Dynamic energy budget theory for metabolic organisation". Cambridge University Press, Cambridge.{{cite journal}}: Cite journal requires |journal= (help)
  21. 1 2 Kearney, M.R. (2022). "What is the status of metabolic theory one century after Pütter invented the von Bertalanffy growth curve?". Biological Reviews. 96 (2): 557–575. doi: 10.1111/brv.12668 . hdl: 11343/275140 . PMID   33205617.
  22. ↑ Kearney, M.R., Domingos, T., Nisbet, R. (2014). "Dynamic Energy Budget Theory: An efficient and general theory for ecology". BioScience. 65 (4): 341. doi: 10.1093/biosci/biv013 .{{cite journal}}: CS1 maint: multiple names: authors list (link)
  23. 1 2 3 Banavar, J. R., Maritan, A., & Rinaldo, A. (1999). "Size and form in efficient transportation networks". Nature. 399 (6732): 130–132. Bibcode:1999Natur.399..130B. doi:10.1038/20144. PMID   10335841. S2CID   204993057.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  24. 1 2 West, G.B., Brown, J.H., & Enquist, B.J. (1999). "The fourth dimension of life: Fractal geometry and allometric scaling of organisms". Science. 284 (5420): 1677–9. Bibcode:1999Sci...284.1677W. doi:10.1126/science.284.5420.1677. PMID   10356399. S2CID   29451718.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  25. 1 2 Banavar, J. R., Damuth, J., Maritan, A., & Rinaldo, A. (2002). "Supply-demand balance and metabolic scaling". Proceedings of the National Academy of Sciences. 99 (16): 10506–10509. Bibcode:2002PNAS...9910506B. doi: 10.1073/pnas.162216899 . PMC   124956 . PMID   12149461.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  26. ↑ Rinaldo, A., Rigon, R., Banavar, J. R., Maritan, A., & Rodriguez-Iturbe, I. (2014). "Evolution and selection of river networks: Statics, dynamics, and complexity". Proceedings of the National Academy of Sciences. 111 (7): 2417–2424. Bibcode:2014PNAS..111.2417R. doi: 10.1073/pnas.1322700111 . PMC   3932906 . PMID   24550264.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  27. 1 2 3 Savage V.M.; Gillooly J.F.; Brown J.H.; West G.B.; Charnov E.L. (2004). "Effects of body size and temperature on population growth". American Naturalist. 163 (3): 429–441. doi:10.1086/381872. PMID   15026978. S2CID   4693534.
  28. ↑ Enquist, B. J., West, G. B., Charnov, E. L., & Brown, J. H. (1999). "Allometric scaling of production and life-history variation in vascular plants". Nature. 401 (6756): 907–911. Bibcode:1999Natur.401..907E. doi:10.1038/44819. S2CID   4397261.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  29. ↑ Enrique Cadenas; Lester Packer, eds. (1999). Understanding the process of ages : the roles of mitochondria, free radicals, and antioxidants. New York: Marcel Dekker. ISBN   0-8247-1723-6.
  30. ↑ Denney N.H., Jennings S. & Reynolds J.D. (2002). "Life history correlates of maximum population growth rates in marine fishes". Proceedings of the Royal Society of London B. 269 (1506): 2229–37. doi:10.1098/rspb.2002.2138. PMC   1691154 . PMID   12427316.
  31. 1 2 Allen A.P., Brown J.H. & Gillooly J.F. (2002). "Global biodiversity, biochemical kinetics, and the energetic-equivalence rule". Science. 297 (5586): 1545–8. Bibcode:2002Sci...297.1545A. doi:10.1126/science.1072380. PMID   12202828. S2CID   131587862.
  32. ↑ Enquist, Brian J.; Brown, James H.; West, Geoffrey B. (1998). "Allometric scaling of plant energetics and population density". Nature. 395 (6698): 163–165. Bibcode:1998Natur.395..163E. doi:10.1038/25977. ISSN   1476-4687. S2CID   204996904.
  33. ↑ Hutchinson, G., MacArthur, R. (1959). "A theoretical ecological model of size distributions among species of animals". Am. Nat. 93 (869): 117–125. doi:10.1086/282063. S2CID   84614449.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  34. ↑ Rohde, K. (1992). "Latitudinal gradients in species-diversity: the search for the primary cause". Oikos. 65 (3): 514–527. doi:10.2307/3545569. JSTOR   3545569. S2CID   40145348.
  35. ↑ Gillooly, J.F., Allen, A.P., West, G.B., & Brown, J.H. (2005). "The rate of DNA evolution: Effects of body size and temperature on the molecular clock". Proc Natl Acad Sci U S A. 102 (1): 140–5. Bibcode:2005PNAS..102..140G. doi: 10.1073/pnas.0407735101 . PMC   544068 . PMID   15618408.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  36. ↑ Terribile, L.C., & Diniz-Filho, J.A.F. (2009). "Spatial patterns of species richness in New World coral snakes and the metabolic theory of ecology". Acta Oecologica. 35 (2): 163–173. Bibcode:2009AcO....35..163T. doi:10.1016/j.actao.2008.09.006.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  37. ↑ Stegen, J. C., Enquist, B. J., & Ferriere, R. (2009). "Advancing the metabolic theory of biodiversity". Ecology Letters. 12 (10): 1001–1015. doi: 10.1111/j.1461-0248.2009.01358.x . PMID   19747180. S2CID   15388080.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  38. 1 2 Michaletz, S. T., Cheng, D., Kerkhoff, A. J., & Enquist, B. J. (2014). "Convergence of terrestrial plant production across global climate gradients". Nature. 512 (39): 39–44. Bibcode:2014Natur.512...39M. doi:10.1038/nature13470. PMID   25043056. S2CID   4463144.{{cite journal}}: CS1 maint: multiple names: authors list (link)
  39. ↑ Banse K. & Mosher S. (1980). "Adult body mass and annual production/biomass relationships of field populations". Ecol. Monogr. 50 (3): 355–379. doi:10.2307/2937256. JSTOR   2937256.
  40. ↑ Ernest S.K.M.; Enquist B.J.; Brown J.H.; Charnov E.L.; Gillooly J.F.; Savage V.M.; White E.P.; Smith F.A.; Hadly E.A.; Haskell J.P.; Lyons S.K.; Maurer B.A.; Niklas K.J.; Tiffney B. (2003). "Thermodynamic and metabolic effects on the scaling of production and population energy use". Ecology Letters. 6 (11): 990–5. doi:10.1046/j.1461-0248.2003.00526.x. S2CID   21068287.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.